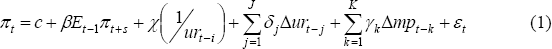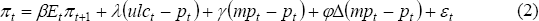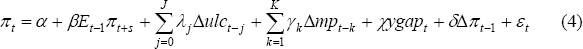RDP 2010-03: Modelling Inflation in Australia 2. Modelling Structure and Theory

The literature identifies many ways to specify an inflation model. Setting aside the multi-equation and factor approaches discussed above, the structure of a single-equation inflation model can differ based on the variables assumed to drive inflation (most prominently, whether to use the output gap, the unemployment rate or marginal costs), how to model inflation expectations and whether to include foreign variables, among other considerations. Given this, we present variants of three types of inflation equations in this paper – both ‘standard’ and New-Keynesian Phillips curve models, and a mark-up model.

2.1 The ‘Standard’ or Expectations-augmented Phillips Curve

One category of inflation model is commonly referred to as the ‘standard’, or ‘expectations-augmented Phillips curve’ model. The original foundation for such models is the framework developed by Phillips (1958), which relates inflation to past and present values of some measure of resource utilisation such as the unemployment rate, so that reduced spare capacity contributes to higher inflation. Since the work of Milton Friedman, Edmund Phelps and others in the late 1960s and 1970s, it has been standard to augment this univariate relationship with inflation expectations, thereby avoiding the implication that there is a long-run trade-off between lower unemployment and higher inflation. Such models have been the workhorse of inflation modelling in a number of central banks over the past three decades.

Empirical estimates of the standard Phillips curve can be found in a multitude of papers. One fairly standard specification relates inflation to several years of lagged inflation (as a proxy for inflation expectations), the current unemployment gap and measures of recent supply shocks (such as the relative price of food, energy and imports). Examples of this type of model include Gordon (2005) and Brayton, Roberts and Williams (1999). A slightly different approach can be seen in papers such as Gruen, Pagan and Thompson (1999), which include a direct estimate of inflation expectations derived from the bond market.

Our specification follows this latter approach by directly including (bond market) inflation expectations. We also including the change in the unemployment rate to reflect ‘speed limit’ terms, and allow for only one supply shock – import price growth. However, our approach differs from Gruen et al (1999) in two respects. First, we do not allow for a time-varying non-accelerating inflation rate of unemployment (NAIRU) (although we assess the relevance of this simplification in Section 6). Second, we do not constrain our results to ensure a vertical long-run Phillips curve (although we consider the impact of this choice in Section 5.1). This results in the following standard Phillips curve model:

Where: π, ur and mp represents inflation, the unemployment rate and import prices, respectively; Δ is the one-period change operator; and E_t−1πt+s represents expectations of inflation over the next s periods, formed in period t−1.

2.2 The New-Keynesian Phillips Curve

A second type of model that is widely used is the New-Keynesian Phillips curve (NKPC; see Galí and Gertler 1999, among others). This builds on the expectations-augmented Phillips curve by explicitly deriving the model from microeconomic relationships between capacity utilisation, costs, prices and nominal rigidities at the firm level. The resulting inflation equation then links the deviation of inflation from its expected level to either the output gap or real marginal cost.

The derivation of the closed economy version of the NKPC is now standard; interested readers should consult Galí (2008) for details. In an open economy setting, the derivation is similar except that it is appropriate to view consumer price inflation as determined by a weighted average of domestic and imported inflation, with the latter equal to the change in real import prices and the former determined as per the standard closed economy NKPC, but with marginal cost proxied by both real unit labour costs and real import prices.^[1] The resulting open economy NKPC can then be expressed as:

where: ulc and mp represent nominal unit labour costs and import prices (world prices, converted into domestic currency), respectively; p is the consumer price level; and all variables are expressed as deviations from steady state. In empirical work it is now also relatively standard to include a backward-looking inflation term in the NKPC to produce a ‘hybrid’ NKPC, drawing on the work of Fuhrer and Moore (1995).

An alternative specification is to use a measure of the output gap as a proxy for real unit labour costs, given that these are proportional under certain conditions (most notably that the capital stock is exogenously determined). However, it has been a point of considerable controversy as to whether including the output gap – defined as the deviation of actual GDP from its potential level – as the driving variable for inflation provides a better representation than other proxies for real marginal cost.^[2] This may reflect the possibility that either the output gap is too imperfectly measured to be a useful proxy for real unit labour costs, or that the conditions under which they are proportional do not hold. We take an agnostic view on this debate and include both the output gap and real unit labour costs in our estimated equations. Theoretically this can be motivated by allowing the output gap to influence inflation (over and above its influence on unit labour costs) via either the cyclicality of non-wage costs (as in Leith and Malley 2007) or a procyclical effect on the mark-up of price over marginal cost (that is, firms may raise their mark-up over marginal cost when demand for their products is high).

As a result, our NKPC model can be specified as:

where: rulc, rmp and ygap are real unit labour costs, real import prices and the output gap, respectively; and all variables are expressed as deviations from their sample means.

2.3 The Mark-up Model

Much of the previous Australian work on inflation modelling has tended to focus on mark-up models of inflation rather than the Phillips curve approach (see, for example, de Brouwer and Ericsson 1995, Heath, Roberts and Bulman 2004 and Bårdsen, Hurn and McHugh 2007). In this model, inflation is determined by current and lagged growth in unit labour costs and import prices, based on the theory that firms set their prices as a mark-up on costs, as in the NKPC. However, there has previously been little explicit allowance for forward-looking behaviour by firms in such models, and the presence of nominal rigidities has only been included implicitly by allowing for lags of input costs.

Nonetheless, it is straightforward to show that the NKPC can be rearranged to reflect an expectations-augmented mark-up model. Full details of this are available in Appendix A, but in short, this involves rearranging the NKPC so that it includes terms for the growth in nominal marginal costs. We also allow for the mark-up to be time-varying and positively related to the output gap. Given no change in the inflation target, this results in the following specification for inflation:

where the notation is as per Equations (1) and (3).^[3]

Footnotes

The inclusion of both the level and change in real import prices allows for a general specification, whereby imports can be either consumption or intermediate goods. [1]

Key players in this debate are Galí, Gertler and López-Salido (2005), who argue in favour of the marginal cost-based NKPC, and Rudd and Whelan (2005), who argue in favour of the (flex-price) output gap-based NKPC. [2]

Bårdsen et al (2007) argue that prices and real wages should be modelled as a system, to reflect the fact that wage growth is dependent on prices and hence endogenous in Equation (4). However, this should only be a serious econometric problem if the contemporaneous correlation is significant, which does not appear to be the case. [3]