RDP 2015-15: Household Economic Inequality in Australia 3. Stylised Facts About Household Economic Inequality

3.1 Long-run Trends in Consumption and Income Inequality

There are several different indicators of inequality that are typically used in the literature. The most common measure of inequality is the Gini coefficient, which is derived from the Lorenz curve. The Lorenz curve shows the share of spending (or income) by households ranked by spending (or income). The further the curve is below the 45 degree line, the less equal the distribution. Correspondingly, the Gini coefficient is calculated as the area between the Lorenz curve and the 45 degree line divided by the total area under the 45 degree line. The Gini coefficient ranges from zero to one, where zero represents perfect equality and one represents complete inequality.

Based on the 2009/10 HES, the Lorenz curve for gross income indicates that the top 20 per cent of households earned approximately 42 per cent of total household income (Figure 1). In contrast, the bottom 20 per cent of households earned about 7 per cent of income. However, income inequality is reduced to some extent by the redistribution of income from rich households to poor households through government taxes and transfers. As a result, in 2009/10 the Gini coefficient for disposable income (0.32) was lower than for gross income (0.35).

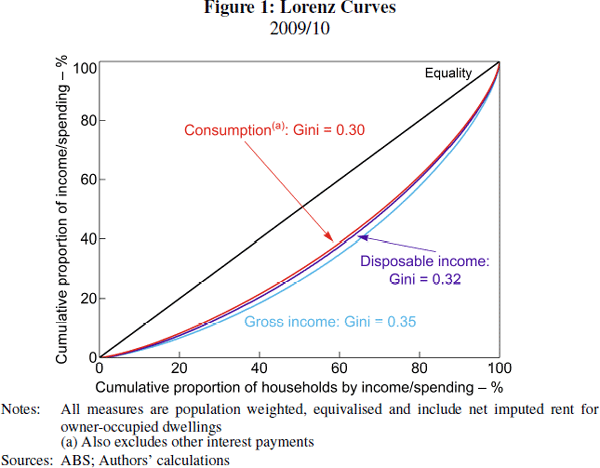
In addition, the Gini coefficient for consumption (0.30) was lower than that of disposable income (0.32), suggesting that economic inequality is further reduced by the ability of households to borrow and save to offset temporary changes in income. The Lorenz curve for consumption indicates that the highest-spending households (in the top 20 per cent) accounted for approximately 39 per cent of total spending in the economy. The lowest-spending households (in the bottom 20 per cent) accounted for about 8 per cent of total spending.

Next, we examine how inequality in both consumption and income has evolved over recent decades. Based on the Gini coefficient, gross income inequality is a bit higher than in the early 1990s, although it moderated in the early 2000s (Figure 2).

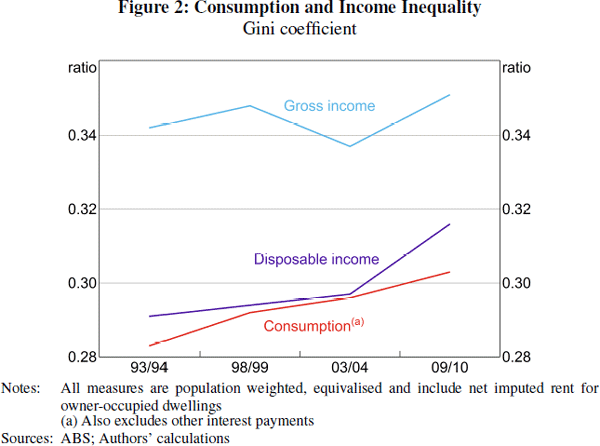
In contrast, the HES estimates of disposable income inequality have risen consistently since the early 1990s. This finding is consistent with other studies and largely reflects an increase in capital income inequality, as labour income inequality has been little changed over recent decades (Greenville et al 2013). According to Greenville et al, labour income inequality has been little changed over the past two decades due to two offsetting effects. On the one hand, high-income households have benefited relatively more from rising hourly wages for full-time employees and an increase in the share of part-time employment (which have tended to increase inequality since higher-income households are more likely to be double-income households). On the other hand, low-income households have benefited relatively more from the reduction in the share of jobless households (which has tended to reduce inequality), which is consistent with the substantial trend decline in the unemployment rate since the early 1990s.

Consumption inequality has been consistently lower than both gross and disposable income inequality. Furthermore, the increase in consumption inequality has also been less pronounced than the increase in disposable income inequality since the early 1990s. In Section 4 we show that these trends have also been observed in other advanced economies. We explore drivers of these changes in the next section of the paper.

The Gini coefficient is a useful indicator for summarising distributions. However, it does not identify which parts of the distribution are responsible for any changes over time. It is also not a particularly intuitive measure of inequality. To complement the analysis, we examine how much of aggregate household income is earned by the high-income households (as a proxy for income inequality) and, similarly, how much of aggregate household consumption is accounted for by the high-spending households (for consumption inequality).

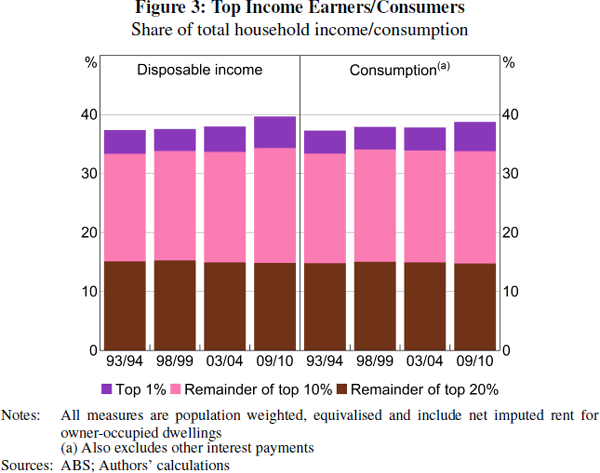
Based on the disposable income estimates, the top 10 per cent of income earners accounted for 22.3 per cent of aggregate household income in 1993/94 and 24.8 per cent in 2009/10 (Figure 3). Much of the increase in the share of income held by the top 10 per cent of earners has been due to the very highest earners within the top 1 per cent – their share of total disposable income rose from 4 per cent in 1993/94 to 5.4 per cent in 2009/10.^[10]

Based on the consumption estimates, the top 10 per cent of spenders accounted for 22.5 per cent of aggregate household consumption in 1993/94 and 24 per cent in 2009/10. Again, a substantial part of the increase in the share of consumption for the top 10 per cent is due to the very biggest spenders within the top 1 per cent – their share of aggregate consumption rose from 3.9 per cent in 1993/94 to 5 per cent in 2009/10.

To further examine different parts of the income and consumption distributions, we break down each distribution into deciles and separate out the top 1 per cent. We then examine the relative growth in income and consumption for each decile and the top 1 per cent. Based on this, the top 1 per cent of earners experienced a relatively large increase in real disposable income of just over 5 per cent per annum between 1993/94 and 2009/10 (Figure 4). In contrast, the bottom 99 per cent of households experienced real annualised income growth of about 3 per cent, with the bottom decile experiencing much lower growth than the rest of the distribution. The top 1 per cent of spenders have also experienced faster growth in real consumption than other households over recent decades, though the difference in growth is less pronounced than in the case of income (Figure 4). More specifically, the top 1 per cent of spenders experienced real growth in consumption of a bit over 4 per cent. In contrast, households in the bottom 99 per cent of the consumption distribution experienced real average annualised growth of about 2.5 per cent, with the bottom decile experiencing growth of just 1.6 per cent.

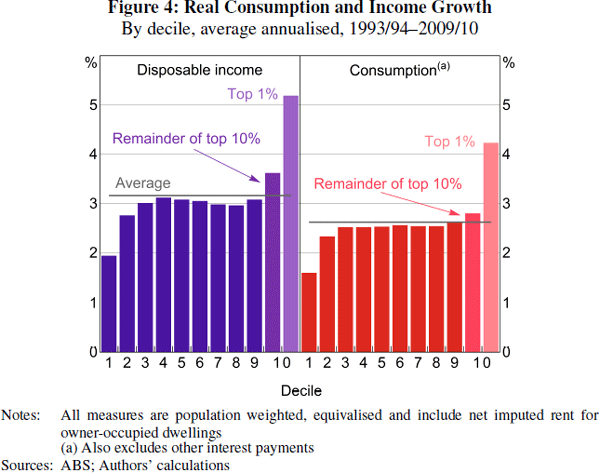
An interpretation of the differing trends in income and consumption inequality is that some of the increase in income inequality has been due to an increase in the variance of transitory income shocks, which households have been able to smooth through borrowing and saving. This is consistent with the permanent income hypothesis, which postulates that consumers spend in line with their permanent income and borrow and save to offset temporary fluctuations in income. We explore the separate trends in permanent and transitory income in Section 5.

3.2 Wealth Inequality

We mainly focus on consumption and income inequality because we have long-run estimates for these measures of household wellbeing. But we also briefly consider wealth inequality for two reasons. First, wealth is a potentially important indicator of wellbeing in its own right. Second, it highlights the important role of housing prices in affecting measured inequality. To develop the most complete picture we consider estimates of wealth inequality from both the HES and SIH.

Wealth is much more concentrated within the household population than either income or consumption. In 2013/14 the Gini coefficient for net wealth was 0.60, which was well above the level of inequality in disposable income (Figure 5). Other indicators of wealth inequality tell essentially the same story. For instance, the share of total net wealth held by the top 20 per cent of wealthy households was 62 per cent in 2013/14. This is 1.5 times the corresponding share of aggregate income for the top 20 per cent of income-earning households.^[11] It is common in both Australia and other advanced economies to find that wealth is much more skewed towards the top of the distribution than either income or consumption.

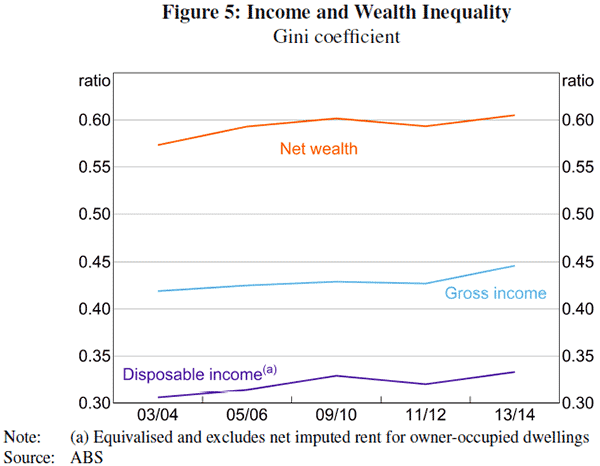
Wealth inequality has risen modestly in Australia over the past decade or so by about the same amount as income inequality. The rise in wealth inequality has been due to changes at both the top and bottom of the distribution. Between 2003/04 and 2013/14, the net wealth of the top wealth quintile rose at an annualised rate of 3.3 per cent, while the net wealth of the bottom wealth quintile increased by an annualised rate of 1.1 per cent. Consistent with this, the share of total net wealth accounted for by the top wealth quintile rose from 59 per cent in 2003/04 to 62 per cent in 2013/14 (Figure 6).^[12]

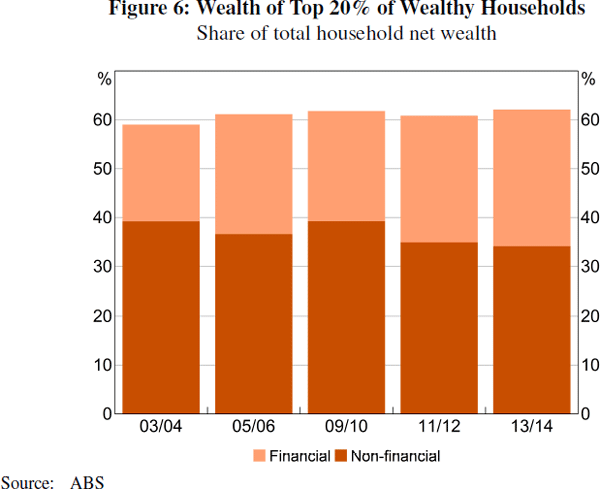
As in most advanced economies, housing wealth is the largest component of aggregate household wealth in Australia. Somewhat surprisingly though, changes in housing prices have not been the main determinants of changes in wealth inequality over the past decade. Instead, the rise in wealth inequality has been due to a rise in inequality in financial wealth and, in particular, an increase in the value of holdings of private superannuation and debt instruments (such as debentures and bonds) for the wealthiest households.

3.3 Housing Prices, Imputed Rent and Inequality Estimates

The estimates of household consumption and income inequality presented in the previous section include a service-flow equivalent of housing expenditure for owner-occupiers (or ‘net imputed rent’). It is worth discussing the adjustment for net imputed rent in detail as it has a significant effect on estimates of both the level and cross-sectional distribution of consumption and income in the economy.

The household surveys show that the inclusion of net imputed rent significantly reduces the level of inequality in both income and spending. Based on the Gini coefficient, the addition of net imputed rent reduces measured inequality in spending by a bit over 6 per cent, on average. This is shown by the fact that total consumption is more equally distributed across households than goods and services expenditure, on average (Figure 7). (Recall that household ‘consumption’ is the sum of household ‘expenditure’ and net imputed rent.) Similarly, the addition of net imputed rent reduces inequality in disposable income by just under 5 per cent on average.

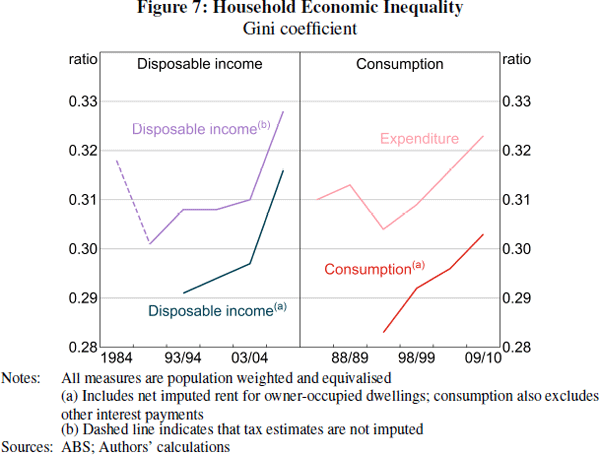
The inclusion of net imputed rent has an equalising effect as it disproportionately benefits low-income (and low-spending) households. This is because the home is typically the largest asset for these households, and as a result, the net imputed rent paid (and earned) on that asset is a relatively large fraction of the household's budget. For example, older (retired) households are likely to have a relatively low level of income (and spending), but a large proportion of these households own their own home outright and, therefore, adding net imputed rent to their measured income (and expenditure) significantly improves their welfare position. On the other hand, many high-income (and high-spending) households are comprised of young renters, meaning that the top of the distribution will not benefit to the same extent by the inclusion of imputed rent in measured income and expenditure.

Essentially, the equalising effect of imputed rent on income is due to the fact that low-income households are not the same as low-wealth households. Net housing wealth (or housing equity) is a relatively large share of total net wealth for low-income households (Figure 8). In contrast, housing equity comprises a relatively low share of total net wealth for low-wealth households (Figure 8). Furthermore, the average rate of home ownership is higher amongst low-income households than amongst low-wealth households (Figure 8). About one-third of households in the lowest wealth quintile are young renter households that are likely to be credit constrained. But nearly half of the households in the bottom income quintile are older home owners.

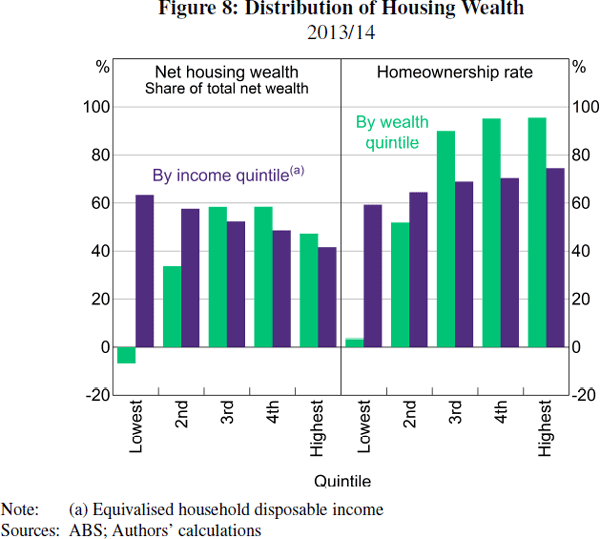
These differences between the income and wealth distributions also imply that changes in housing prices can have different effects on the trends in estimated income and wealth inequality. For instance, if we adjust for net imputed rent, a rise in housing prices can cause estimates of income (and consumption) inequality to fall, all other things being equal, as lower-income owner-occupier households benefit disproportionately from the higher housing prices. At the same time, higher housing prices cause estimated wealth inequality to rise as wealthier households benefit disproportionately.

3.4 Consumption Inequality by Type of Household

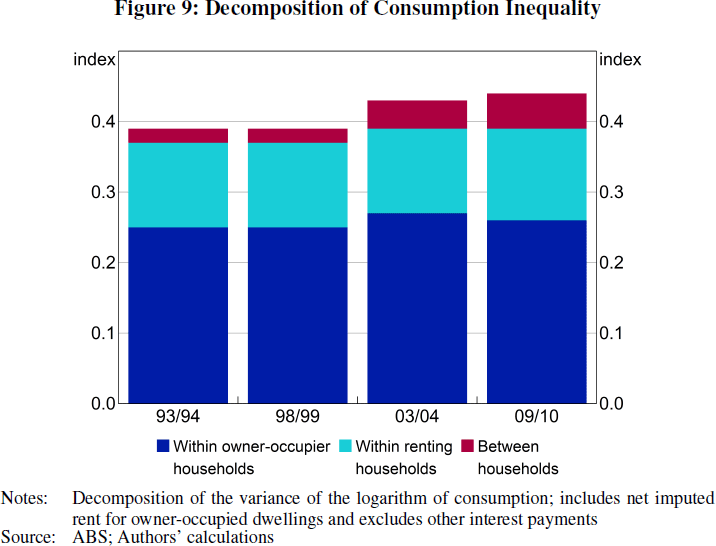
Given the important role of home ownership in affecting measured inequality, it is useful to decompose overall consumption inequality into inequality within both renting households and owner-occupier households as well as inequality between these two groups of households. We do this using analysis of variance (ANOVA); for each survey year, the observed variance in consumption is essentially partitioned into three components: 1) the variance in consumption for owner-occupiers (‘within-owners’); 2) the variance in consumption for renters (‘within-renters’); and 3) the difference between the average level of consumption for owner-occupiers and renters (‘between owners and renters’).^[13]

Based on this, we find that much of the trend increase in consumption inequality over the past decade has been due to a rise in inequality between the two groups (Figure 9). In particular, owner-occupier households consume more goods and services than renter households, on average, and this has become increasingly true over time.

Footnotes

In Appendix C we provide similar estimates of income inequality using tax record data. The consistency between data sources suggests that HES survey data adequately captures top income earners. [10]

The corresponding share of wealth held by the top 1 per cent was about 13 per cent. [11]

The share of wealth held by the top 1 per cent of households follows a similar pattern, rising from 12 per cent in 2003/04 to 13.4 per cent in 2013/14. [12]

More specifically, we decompose the overall variance of log consumption at a point in time as:

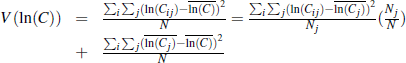[13]
View MathML

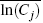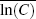where i denotes households and j denotes the number of household groups (in this case, there are two groups – renters and owner-occupiers). Also, = ∑_i ln(C_ij)/N_j denotes the average level of log consumption within each household group, = ∑_i ∑_j ln(C_ij)/N denotes the average level of log consumption across all households, N_j denotes the total number of households within each group while N denotes the total number of households. The first component on the right-hand side of the equation is the ‘within-groups’ component. The second component is the ‘between-groups’ component.