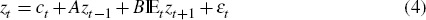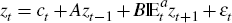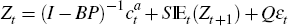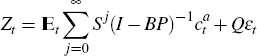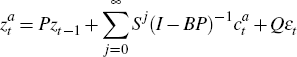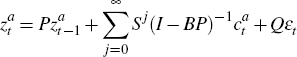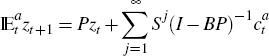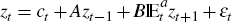RDP 2013-11: Issues in Estimating New-Keynesian Phillips Curves in the Presence of Unknown Structural Change 3. A Simple Variant of the Solution Algorithm
September 2013 – ISSN 1320-7229 (Print), ISSN 1448-5109 (Online)
- Download the Paper 587KB
Kulish and Pagan (2012) set out an algorithm that can be used to compute solutions to a system in which there are changes in structural parameters that are potentially foreseen. It has more general application than the context we are working with here, so we provide a simplified discussion of its workings to highlight some of its significant features.
The system we consider has the format:
where zt is a vector of n (=3) variables (πt,
xt, rt),
ct are the intercepts in the equations, and εt
are identically and independently distributed (iid) (0,σ2)
shocks. It is necessary to allow agents to have different beliefs about the
timing of any breaks than is the case in reality. To study the effects of mean
breaks we assume that agents always know the true shocks and the parameters
A and B of the system, and that their beliefs may only be
incorrect about ct. Agents will be assumed to believe that
the intercepts of the three equations at time
t have the values
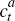 rather than ct.
Then agents solve the system in Equation (4) (with
rather than ct.
Then agents solve the system in Equation (4) (with
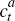 replacing ct) to
form their expectations
replacing ct) to
form their expectations
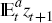 , where the ‘a’ indexes
the agent's beliefs. Those expectations will then determine the actual
outcomes of the system, i.e. the observed variables will be consistent with:
, where the ‘a’ indexes
the agent's beliefs. Those expectations will then determine the actual
outcomes of the system, i.e. the observed variables will be consistent with:
We adopt the Binder and Pesaran (1995) solution method to solve the system. Briefly,
this involves converting Equation (4) into a purely forward-looking form, solving
that, and then recovering the solution to the original system. In the case
that agents believe that the economy is described by Equation (4), with
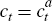 the solution method comes down to solving
the solution method comes down to solving
where Zt = zt − Pzt−1, S = (I − BP)−1B, Q = (I − BP)−1T and P is chosen such that (A+BP2−P) = 0. Because shocks are iid, the solution will be
Now we can write the complete solution in one of two forms – either
or
In the first case, agents compute the weights to be applied to zt−1 from what they believe the data-generating
process
(DGP)
for the macroeconomic variables is, i.e. actual past outcomes are
used in forming expectations. In the second, they solve for the complete path
that they would have expected if the DGP was from the model compatible with
their beliefs. The former seems more reasonable as the latter implies that
agents persist in believing in a path even when they can observe persistent
departures from it. Up until the break there will be no difference, but thereafter,
unless the agents know exactly when the break occurs, there will be a systematic
difference between zt−1 and
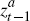 , since zt will
adjust to the structural changes when they happen. In ‘control’
jargon the path
, since zt will
adjust to the structural changes when they happen. In ‘control’
jargon the path
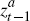 is often called the ‘open loop
controller’ while that based on zt−1
is the ‘closed loop controller’. Our algorithm will allow for expectations
formation based on either zt−1 or
is often called the ‘open loop
controller’ while that based on zt−1
is the ‘closed loop controller’. Our algorithm will allow for expectations
formation based on either zt−1 or
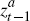 .
.
In the first case, expectations formation would follow
and the values of zt that are generated by the model economy will then be
The solution for the second case is analogous to this.