Research Discussion Paper – RDP 2019-10 Emergency Liquidity Injections
1. Introduction
If financial intermediaries in liquidity distress are to be bailed out, how should it be done? In late 2008 policymakers were forced to decide quickly. The world's largest banking systems experienced a rapid decline of funding liquidity, alongside fire sales and a drying up of market liquidity for many securities. Financial intermediaries (henceforth ‘banks’) with insufficient cash or high-quality liquid assets (HQLA) then had difficulty meeting their short-term liabilities. Governments and central banks (i.e. authorities) were aware that bailouts could damage banks' incentives to manage liquidity risk (e.g. Bernanke 2008), but nonetheless responded with massive liquidity injections, because avoiding imminent bank failures was a higher priority.
Trillions of US dollars worth of emergency liquidity was provided to banks around the world through a range of policies. The largest provisions were implemented with secured lending (i.e. repo) via central banks' market operations and with government guarantees on banks' unsecured debt issuance. Capital injections and secondary market securities purchases were also relied upon to varying degrees.[1] To prepare policymakers for future crises, a range of research papers have analysed these policies for saving banks, but secured lending, arguably the most-used policy in the 2008 liquidity crisis, has received little comparison with other injection policies. Moreover, policy comparisons tend to emphasise the near-term effects on the financial system, whereas in the longer term, effects on incentives may be just as consequential.
This paper addresses these gaps by considering alternative policies in a banking crisis model that incorporates a range of features from the literature. Banks choose their asset-side liquidity risk by allocating their portfolio across highly liquid and less-liquid securities (e.g. Diamond and Dybvig 1983). The prospect of a funding liquidity shock encourages banks to hold some highly liquid securities (Hanson et al 2015), but they hold too few due to fire sale externalities (Stein 2012). Subsequently, if there is no funding liquidity shock, the riskier securities pay positive returns; if there is a shock, banks must cover a randomly drawn cash outflow. When banks' liquid securities are insufficient to meet the outflow, they sell their less-liquid securities, which can temporarily put downward pressure on market liquidity and the securities price (e.g. Brunnermeier and Pedersen 2009; Diamond and Rajan 2011). A ‘banking crisis’ eventuates if the price falls to the point that banks cannot handle the liquidity shock, characterised primarily by an inability to liquidate assets at ‘fair’ values. Its probability is endogenous because the maximum manageable liquidity shock is decreasing in banks' liquidity risk-taking (e.g. Goldstein and Pauzner 2005). In a banking crisis, the authority injects scarce liquidity to prevent bank failures, calibrating its policy to maximise its objectives of low ex ante liquidity risk-taking and high ex post bank profits (e.g. Farhi and Tirole 2012).
This relatively simple crisis anatomy yields some important characteristics of liquidity injections that have not been highlighted by previous papers. The main underlying result is that lending to banks against securities as collateral, rather than unsecured, can mitigate fire selling in illiquid securities markets. Both the secured and unsecured lending policies can put an upper bound on fire sale price depression at the interest rate charged, by offering banks a cheaper source of liquidity than securities sales. However, low interest rates on emergency lending also incentivise liquidity risk-taking and make a crisis more probable. Secured lending presents an additional means of constraining fire selling, because banks cannot liquidate securities that they are providing as collateral. In other words, collateral obligations force banks to treat emergency borrowing and securities sales as substitutes. If the lending policy gives banks more liquidity for their securities than sales – by, for example, not pricing fire sale effects into the collateral valuation – then higher liquidity needs imply more borrowing and less selling. That is, the more scarce is liquidity, the greater is the role played by the authority's balance sheet, which is immune to illiquidity, in replacing the liquidity provision function of securities markets.
Ashcraft, Gârleanu and Pedersen (2011) also argue that liquidity injections through secured lending can raise market prices for securities, but they highlight different mechanisms. In their model, the central bank can expand secured lending by reducing its haircuts, which raises securities prices by lowering the cost of funding securities purchases. That is, lower haircuts permit banks to fund a higher proportion of their purchases by borrowing against the purchased securities, and this funding is cheaper for banks than using their own capital. The mechanism can be thought of as a loosening of constraints on banks' demand for securities. In contrast, the model presented here emphasises the policy's capability to tighten constraints on the supply of securities available to fire selling banks. Both models show that low haircuts are beneficial for banks that need liquidity; this paper additionally demonstrates that ideal haircuts are not so low that the policy economically resembles unsecured lending.
Moreover, the authority can better achieve its objectives if its emergency lending is secured rather than unsecured because mitigation of fire sale prices positively affects banks' post-crisis profits. Importantly, this benefit does not raise incentives for liquidity risk-taking. Instead, it offsets fire sale externalities driven by banks' failure to acknowledge how their own securities sales contribute to price depression. The banks that benefit most from higher securities prices are those whose market participation is least constrained by collateral requirements; that is, those that took less liquidity risk and have less emergency borrowing to collateralise. The result is that, compared to an unsecured lending policy, a secured lending policy can leave banks more profitable post-crisis without generating a higher ex ante likelihood of a crisis occurring.
The model presents several additional implications for emergency lending policies. ‘Penalising’ interest rates on emergency lending have been recommended by previous literature for two reasons: (i) to incentivise banks to miminise their borrowing from the authority (e.g. Bagehot 1920); and (ii) to disincentivise banks from taking liquidity risk ex ante (e.g. Fischer 1999). This paper demonstrates an equivalency between the two. Achieving (ii) requires that liquidity risk-taking be costly at the margin, and since the marginal effect of taking liquidity risk is to increase the probability of requiring emergency lending, objective (ii) requires that emergency borrowing is more expensive than private sources of liquidity. The same condition determines which liquidity source banks prefer to exhaust first, tying it to objective (i).
A second implication for emergency lending policies is that secured lending can force banks to borrow more from the authority than unsecured lending, because banks' liquidity acquisition through securities sales is constrained by their need to provide collateral. Relative to an unsecured policy, this amplifies the disincentive effect of penalising interest rates, but also means more expansion of the authority's balance sheet.[2] A third implication is that haircuts under a secured lending policy should be sufficiently loose; specifically, they should permit banks to extract more liquidity from their collateral than they can get in the private market. Otherwise, the policy provides little benefit to banks that face failure due to insufficient access to liquidity (Allen and Carletti (2008) make a similar recommendation). Authorities appear to have followed this principle in 2008 and 2009, by expanding collateral eligibility to securities whose markets had dried up.[3]
Another way the authority can save banks is by buying their securities at a higher price than the private market is willing to pay. The price paid must be generous enough to transfer banks the amount of liquidity they need, so, for a given quantity of securities held by banks, it is increasing in the extent of banks' liquidity deficiency. Accordingly, if banks coordinate on a relatively high level of liquidity risk, then conditional on a crisis they are more liquidity deficient and receive a more favourable policy. Banks' liquidity risk choices are therefore strategic complements. Farhi and Tirole (2012) demonstrate a similar result in a model in which different forms of liquidity injections are equivalent, showing that policies that aim to disincentivise ex ante liquidity risk-taking are not credible. In the model presented here, lending policies that permit banks to repay the loans after conditions improve are more credible than a securities purchase policy.
This paper's portrayal of a liquidity crisis is key to the credibility difference between policies. The core market imperfection is a temporarily limited supply of cash, which causes unexploited arbitrage opportunities in the securities market and an implicit inability for banks to borrow against other non-marketable assets at any interest rate. A similar perspective of liquidity is presented by several of the citations above (Diamond and Rajan 2011; Stein 2012; Hanson et al 2015). A consequence is that banks can benefit from borrowing even if it is costly, because it can save them from failing if they can delay the costly repayment until the availability of cash improves. Alternatively, if banks' access to liquidity is directly tied to the sale price of their marketable assets (as in this paper's securities purchase policy) or determined by the interest rate at which their non-marketable assets can be pledged (as in Farhi and Tirole (2012)), then banks can only be saved by being offered generous terms. The policy implication is that in the type of liquidity crisis this paper models, emergency lending policies, if for sufficiently long terms, can simultaneously provide liquidity support and be penalising, so penalising terms can be credible.
This paper contributes to the literature on crisis interventions by providing an ex ante and ex post analysis of a range of policies for preventing illiquidity-driven bank failures. A variety of insightful papers model crisis interventions, although the focus typically differs in either or both of the following: i) illiquidity-driven bank failures are not possible or not undesirable; or ii) the policy objectives do not include the incentives generated. Acharya and Yorulmazer (2008) and Acharya, Shin and Yorulmazer (2011) show how asset purchase policies can be designed to generate prudent incentives, with an authority that credibly permits bank failures. He and Krishnamurthy (2013) compare the ex post effects of lending, asset purchase and capital injection policies on liquidity-affected asset prices. Farhi and Tirole (2012) and Philippon and Schnabl (2013) consider policies aimed at stimulating bank lending, without the possibility of bank failures. The former compare the ex post implications of system-wide and bank-specific funding subsidisations, and the latter analyse the optimal design for a capital injection policy. Tirole (2012) and Choi, Santos and Yorulmazer (2019) analyse how various policy formulations can jump start frozen asset markets. This paper also contributes to the recent literature on how central banks' collateral frameworks affect banks' securities portfolio decisions (Nyborg 2017; Cassola and Koulischer 2019).
This paper has seven sections. Section 2 presents the model framework. Sections 3, 4 and 5 analyse unsecured lending, secured lending and asset purchase policies, emphasising points of comparison. Section 6 draws insights from extensions that relax some of the model assumptions and considers a policy that injects capital into banks. Section 7 concludes. Appendix A discusses the liquidity injection policies implemented in Europe and the United States in 2008 and 2009. Proofs of the model results are in Appendix B.
2. The Model
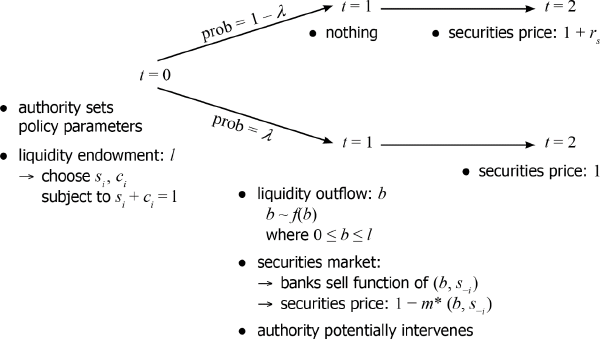
The model has three dates, t = 0, 1, 2, and three types of agents: the authority, a continuum of risk-neutral banks, and securities buyers. The modelling goal is to compare policies by how well the authority can achieve its objectives given banks' reactions to the policy and their interactions with securities buyers. The time line is:
- The authority announces its policy parameters, such as the interest rate on emergency lending, given the type of liquidity injection policy. Banks then choose their asset-side liquidity risk to maximise their expected date 2 pay-off .
- A liquidity shock may occur, whereby banks experience a randomly drawn outflow of cash. Banks can cover a cash shortage by selling securities to securities buyers or by obtaining emergency funds from the authority. The market price for securities equilibrates banks' demand for liquidity via sales with securities buyers' optimal liquidity supply.
- If there was a liquidity shock at date 1, market liquidity and the securities price recover. If there was no liquidity shock, securities pay positive returns.[4]
2.1 Banks and the Liquidity Shock
At date 0 the continuum of risk-neutral banks each have a liquid endowment l. They can allocate l between two types of liquid assets – securities ‘s’ and cash ‘c’. Securities have positive expected returns, but if there is a liquidity shock, market illiquidity can depress their prices. Cash has zero net return but always holds its value. Denote bank i's securities choice by si. The date 0 securities price is normalised to 1 so ci + si = 1 for all i.
Denote the set of choices (i.e. the strategy profile) of a unit measure of banks that excludes bank i as s–i, and the set of all banks' choices as s. This can be thought of as the mapping s : [0, 1] → [0, l], which is assumed non-decreasing and integrable in i. Total securities held by banks is defined as . The statement s–i = S indicates that a unit measure of banks each hold S – i.e. a (virtually) symmetric choice across other banks – and indicates there is a positive measure of banks not choosing S. The statement s = S indicates fully symmetric choices at S.
At date 1, with probability , where , nothing happens and at date 2 securities are worth 1 + rs > 1. With probability there is a liquidity shock and every bank must provide cash b, drawn randomly from distribution f (b) which is positive and continuous on [0, l). The shock b can be interpreted as short-term creditors not rolling over debt, withdrawing depositors, credit line drawdowns, or unexpected margin requirements.
2.1.1 Discussion
The investment decision resembles the classic portfolio decision in Diamond and Dybvig (1983). Cash ci represents cash and highly liquid low-return securities such as government bonds, whereas securities can be thought of as relatively safe privately issued debt. Banks' other non-marketable assets are treated as outside this liquidity management decision. The simplifying dichotomy resembles how liquidity risk management is interpreted by the liquidity coverage ratio (LCR) of Basel III, which requires banks to hold a sufficient quantity of high-quality assets with low market liquidity risk, distinguishing them from lower quality securities.[5] It is also motivated by the fact that banks had high exposures to assets with markets that were liquid before the crisis, but became illiquid during the crisis, as documented with respect to private secured lending markets by Hordähl and King (2008) and Gorton and Metrick (2012), and with respect to asset-backed securities markets by, for example, Brunnermeier (2009).
2.2 Securities Buyers and the Date 1 Securities Market
Given a liquidity shock at date 1, if ci < b then bank i must satisfy its cash shortage by selling securities or borrowing from the authority. Banks can sell securities to securities buyers or other banks with spare cash (although the paper focuses on symmetric bank choices). For now, assume that bank i only borrows from the authority any shortage it cannot meet with its cash and securities. This assumption permits the authority's behaviour to be described separately; it is relaxed in Section 2.4.
Securities buyers maximise profit by allocating their limited cash across securities purchases and an outside investment option. The outside investment has continuously decreasing net returns that are bounded and equal zero when all their cash is invested. Securities buyers are assumed capable of short selling, which prevents prices rising above 1, and have at least l cash, so can buy all securities held by banks if the returns are high enough. Given date 1 securities price 1 − m, securities buyers' optimal expenditure on securities (LS) therefore satisfies:
where and is positive and continuous. The market-clearing securities price is written 1 – m* where m* represents market illiquidity. The characteristics of securities buyers imply for any demand schedule.
Banks' total demand for liquidity from the securities market, in cash value, is written LD. Denote the quantity of securities sold by bank i as sim, and the total quantity of securities sold by banks as Sm, implying that . For example, if b > ci for all i and no bank needs to borrow from the authority, then sim (1 – m) = b – ci and
Date 1 securities market equilibrium requires that m* satisfies
where b is exogenous, and other variables in LD are determined at date 0.
Figure 1 illustrates m* for two different forms of LD (which correspond to the functional form in Equation (5) later in this section). When banks have spare cash, so , market illiquidity m* is zero, illustrated by and . If LD(m) > 0 then securities buyers buy securities from banks, and m* is where the price is low enough that securities buyers are willing to divert sufficient funds from their outside option to meet the liquidity demand. This is illustrated by and .
Market illiquidity m* is an implicit function and in some cases can be more intuitively expressed as the inverse of the Ls function. This is denoted M such that . The characteristics of LS imply that M (LD) = 0 for all , and that the first derivative of M, written M′, is continuous and positive for all LD > 0. The general expression for equilibrium market illiquidity used throughout this paper, given policy P, is , acknowledging that banks' date 1 securities selling Sm is determined by b and their date 0 securities choices s–i. Sometimes the P subscript will be dropped if the policy type is obvious or irrelevant.
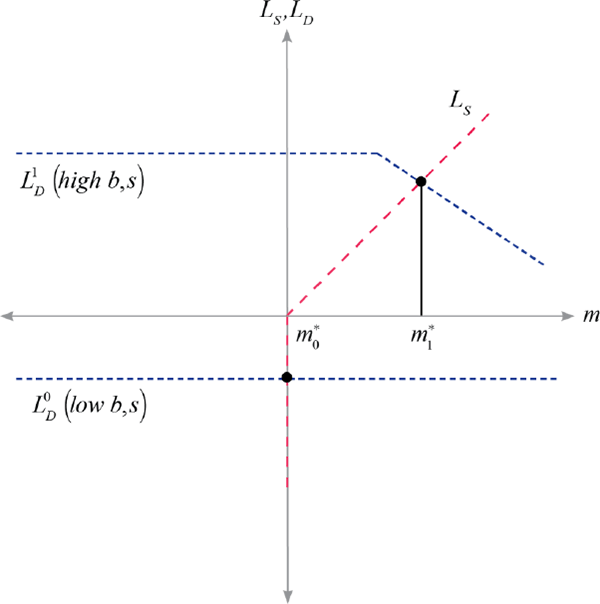
Notes: Illustrates equilibrium securities market illiquidity m*, corresponding to equilibrium price 1 – m*, as the intersection between supply of liquidity from securities buyers and demand for liquidity by banks. LS is a representation of Equation (1). The two schedules of LD are consistent with Equation (5), with representing higher b and/or s than . The kink in reflects the point at which banks are selling all their securities. The axis lines are at zero.
The date 1 value of bank i's liquid assets – its cash and securities prior to any borrowing from the authority – is ci + si (1 – m*) = l – sim*. Therefore, when it does not borrow from the authority, its date 1 liquidity position is l – sim* –b. The liquidity shock that would expend precisely all of bank i's liquid assets is written . Specifically, the implicit function is defined by
If then bank i can meet its cash shortage by selling securities. Banks are assumed to buy at date 1 if they have spare cash and are indifferent between buying and selling. If then bank i is liquidity deficient and must obtain funds from the authority. Given the temporary assumption that banks minimise borrowing from the authority, if s = S (i.e. symmetry) then market illiquidity takes the form
2.2.1 Discussion
Securities market illiquidity is the key market imperfection in the model, caused by bounds on private entities' supply of cash. Banks would never become distressed if securities could always be liquidated at net present value. The market clearing mechanism is similar to Diamond and Rajan (2011) and Stein (2012). It is a generalisation of the cash-in-the-market pricing of Allen and Gale (1994), such that the cash available for securities purchases continuously increases as the market price falls. Ample liquidity returns to the market at a later date, bringing the securities price back to its net present value. Liquidity-driven selling can therefore push prices below their (discounted) future value, consistent with empirical studies of securities prices such as Coval and Stafford (2007), Hameed, Kang and Viswanathan (2010), Longstaff (2010) and Merrill et al (2014).
Funding liquidity and market liquidity are linked by the constraint that if a bank's outflow of short-term liabilities cannot be funded by cash, it must be met by liquidating assets. This link is why the LCR requires banks to hold a quantity of HQLA that depends on their short-term liabilities. It is empirically documented by Nyborg and Östberg (2014), who term such securities liquidation as ‘liquidity pullback’, and by Fontaine and Garcia (2012). This model feature resembles the ‘liquidity spiral’ in Brunnermeier and Pedersen (2009), whereby to meet a tightened capital constraint, investors must sell assets, pushing down the asset price and further reducing the value of their capital.
2.3 An Example of m* and
Consider the market illiquidity function
where . Assume that s = S and market illiquidity is consistent with Equation (5). Symmetry implies that is the same for all banks; denote this . Market illiquidity satisfies
From Equation (4), the threshold can be solved as
Figure 2 plots the market illiquidity function (Equation (6)) for two fixed values of S such that 0 <S′ < S″ < l. Given S, for b below the first kink (on the x axis), at b = l – S, banks' net securities sales are zero and there is no market illiquidity, as is the case for in Figure 1. Above the second kink at , banks are liquidity deficient and selling all their securities to securities buyers, so m* is flat. When S increases, market illiquidity rises at all b > l – S and both kinks shift left.
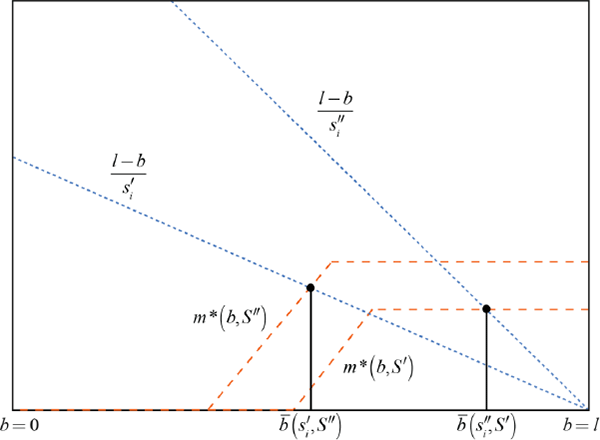
Notes: Represents as the intersection between: (i) market illiquidity m*, which is increasing in b as described in Section 2.2; and (ii) the bank's ability to handle market illiquidity, which is decreasing in b because the higher the liquidity shock, the more liquidity the bank needs to obtain through securities sales. Market illiquidity m* is as characterised in Equation (5) with and . Securities holdings satisfy
Also shown is bank i's capacity to survive market illiquidity (l – b)/si for two fixed values of si such that . The intersection of m* and (l – b)/si determines because bank i avoids liquidity deficiency if and only if . For any si > 0, at b = l bank i cannot survive any market illiquidity because the liquidity withdrawal requires its full date 0 liquid endowment. An increase in si pivots bank i's illiquidity capacity anticlockwise around (l, 0), lowering its and reducing its probability of avoiding liquidity deficiency for any given S.
2.4 The Authority and Equilibrium
The model outcomes are compared across different types of liquidity injection policies P. The authority has full information and, given policy P, announces the policy parameters p (such as the interest rate) at the start of date 0. At date 1, the authority provides liquidity on the terms announced. Banks always accept a liquidity injection rather than failing.[6] Figure 3 summarises the time line of events.
The authority sets the policy parameters to maximise its objective W, defined on: whether there are any bank failures banks' liquidity risk (S), which maps directly to the probability of an intervention being required; and banks' expected profits , which represents ex post banking system health. Preventing bank failures is prioritised above all else, but given this, no particular weighting is assumed on the other two objectives. Specifically, the authority maximises the objective function
where w1 is a positive constant such that w1 > maxp{w2}, and w2 satisfies and
The authority is assumed to prioritise the objectives embedded in W over any effects on its own balance sheet. This objective function represents the view that, during a severe crisis, profits and balance sheet exposure are less of a concern to the authority than the health of the financial system. Consistent with this view, the crisis in this model involves excess demand for liquidity, so for a liquidity-rich authority, interventions tend to have low risk relative to returns. Indeed, authorities made profits on several of the crisis interventions in 2008 and 2009. Swiss National Bank (2013) writes about its liquidity provisions to UBS: ‘attaining a profit was never an objective in its own right. The prime reason for the establishment of the StabFund was its contribution to strengthening the Swiss financial system’.
The preferences represented by W are assumed time consistent. Otherwise, the authority could announce penalty rates in an attempt to induce low liquidity risk, then at date 1 charge lower rates than it announced, to raise banks' post-crisis profits. Time consistency of this objective function is appropriate for two reasons. First, the too-big-to-fail problem is built directly into the authority's first priority – policies under which banks fail are time inconsistent. Second, penalty rates have the ex post benefit to the authority of deterring excessive use of its balance sheet, which is the justification for penalty rates put forward by Bagehot (1920).
The paper focuses on the following three policies (P, p):
- Unsecured lending (u, ru): the authority lends any quantity of emergency funds to bank i, with repayment at date 2 of ei (1 + ru), where ru > 0.
- Secured lending (also called repo) (R,(rR,h)): the authority lends any quantity of funds to bank i, provided that bank i provides the authority ei/(1 – h) securities as collateral (where h is the haircut), with repayment at date 2 of ei (1 + rR), where rR > 0.
- Securities purchase (S,mS (b)): the authority determines an acceptable schedule of market illiquidity mS (b) and purchases sufficient securities such that the date 1 market price does not fall below 1 – mS(b).
Denote by the pay-off maximising securities holdings for bank i given the choices of other banks and the policy. That is,
An equilibrium (p) is a choice set satisfying for all i, with corresponding aggregate securities holdings written . An optimal equilibrium is an equilibrium in which policy parameters p are set to maximise W; specifically,
The P subscripts will sometimes be dropped when there is no ambiguity.
3. Liquidity Injection through Unsecured Lending
Under an unsecured lending policy, bank i's pay-off function is
subject to the liquidity constraint
and the feasibility constraint
The first term on the right-hand side of Equation (9) captures the value of liquid assets after no liquidity shock (l + sirs). The second term represents the liquidity shock outcome, and has three components: the liquidity endowment minus what is lost to the shock (l – b);[7] the losses (gains) from any securities sales (purchases) in an illiquid market (simm*); and any date 2 repayments to the authority (ei + eiru). The constraint in Equation (10) ensures that bank i's securities liquidation revenue plus its borrowing is at least its cash shortage. The constraint in Equation (11) reflects that bank i cannot sell more securities than it owns.
Securities sales (sim) and borrowing from the authority (ei) are choices made at date 1 that can be expressed as functions of predetermined variables (b,si,s–i,ru). Whenever m* > 0, banks raise only the minimum liquidity required and do so via the cheapest sources available. Raising one unit of liquidity through the securities market requires selling 1/(1 – m*) securities, which would have each had value 1 at date 2. Therefore, borrowing from the authority is more expensive than securities sales if and only if 1 + ru >1/(1 – m*).
Accordingly, if ru is low enough, the lending policy places an upper bound on market liquidity:
The securities market cannot be in equilibrium at higher m* because banks would reduce selling and instead source funds from the authority.[8] If there is some liquidity shock b at which Equation (12) binds, it also binds at all higher b, because market illiquidity m* is weakly increasing in b (see Lemma 9 in Appendix B). Denote by b the lowest b at which Equation (12) binds, so satisfies
It is possible that ru is high enough such that Equation (12) does not bind at any b, and is not defined by the first case in Equation (13). In this case set at b = l for notational convenience. An illustration of how ru can bind is presented by the red dashed lines in Figure 4.
If , banks are indifferent between selling securities and borrowing. Each individual bank's selling is indeterminate, but total securities selling is precisely the quantity that, given b and ru, ensures that Equation (12) binds. Accordingly, bank i's liquidity raised through securities has three cases:
Note that if defined in Equation (4) is above , then does not represent a threshold at which bank i is forced to borrow, and has no particular relevance for bank i.
Bank i meets its remaining liquidity deficit by borrowing from the authority:
Combining Equations (9), (14) and (15), bank i's pay-off, taking ru as given, can be expressed as
The expression l – sim* – b captures bank i's spare liquidity, or liquidity deficit if negative. The expression it is multiplied by – either 1/1(1 – m*) or 1 + ru – is the return on liquidity. This is what would be saved (spent) if bank i held one additional (less) unit of cash. If , banks would raise additional liquidity through the securities market, and the return on liquidity is the date 2 value of the securities that must be sold to raise one unit of cash. If bank i is forced to borrow from the authority, and the return on liquidity is the cost of borrowing 1 + ru. If , then the return on liquidity is 1 + ru = 1/(1 – m*) whether bank i is borrowing out of necessity or choice.
Proposition 1. Among symmetric choice sets s, there is a unique equilibrium . If rs is low enough relative to ru for , then is strictly decreasing in ru, bound below only at zero.
Each bank chooses si by comparing the returns that securities provide in the normal state (rs) against the expected costs given a liquidity shock. These costs depend on how many securities other banks hold, because higher S tends to generate more selling pressure, and more market illiquidity whenever m* > 0. Higher market illiquidity lowers the expected marginal return to securities, because depressed prices raise the cost of selling securities and similarly the value of spare cash. Accordingly, as S increases, securities' expected returns decrease, generating a fixed point where S equals the optimal si given S, so that acting in line with other banks is the best response to their choices. This fixed point is decreasing in ru, which enters negatively into banks' marginal return to securities.
Lemma 2. Under an unsecured lending policy, if the level of liquidity risk S that maximises collective bank pay-offs is interior, banks' equilibrium liquidity risk is higher.
Banks' excessive liquidity risk-taking is a form of fire sale externality, modelled the same way as Stein (2012). Market illiquidity is determined by banks' collective liquidity risk-taking, but individual banks' contributions are small enough that they do not factor this cost into their pay-off maximisation. Chernenko and Sunderam (forthcoming) provide empirical evidence for this type of externality in US securities markets. In this model the collective optimum is more informative than a social planner solution. This is because the authority is assumed to not care about its own profit; its benefit from high interest rates is that they deter risk-taking. A social planner would circumvent this deterrent, resulting in a meaningless optimum of S = 0 with infinite funds transferred from the authority to banks.
As mentioned earlier in this section, if ru is high enough, there is no b such that Equation (12) binds. Denote by rpen the lowest ru that induces an equilibrium with this outcome. The condition ru > rpen aligns with the interest rates on emergency lending that Bagehot (1920) recommends, which have since been termed ‘penalty rates’. When ru > rpen, sourcing liquidity from the authority is necessarily more costly than through the securities market, so banks limit their emergency borrowing to the minimum required to avoid failure. The condition also implies strict concavity of , because raising si lowers , which raises the probability of needing to borrow from the authority and paying penalty rates. Therefore, ru >rpen implies both (i) a declining marginal return to si, and (ii) that banks minimise ei to b + sim* – l.[9]
4. Liquidity Injection through Secured Lending
Under a secured lending policy, when a bank borrows from the authority it must provide securities as collateral, which introduces a constraint on its securities sales. The policy can be interpreted as raising the amount of liquidity that a bank can extract from its securities holdings. A security's ‘liquidity value’ if sold is the illiquid price 1 – m*, and if used as collateral is 1 – h where h is the haircut set by the authority. If banks would fail without an intervention, then h < m* is a necessary condition for the policy to avert bank failures. For tractability the following assumes that the authority sets h = 0, which is the lowest non-negative haircut that eliminates its counterparty risk, and which also permits the simplification that h does not depend on b (from the requirement that h < m*). The assumption that h = 0 implies securities retain their full liquidity value if used as collateral, which is discussed later in this section.
The secured lending policy is characterised by Equation (9) (after replacing ru with rR) and Equation (10), but the feasibility constraint Equation (11) is replaced by the collateral constraint:[10]
The collateral constraint does not bind if: (i) bank i has spare liquidity or (ii) borrowing from the authority is not more costly than selling securities , in which case bank i can maximise its pay-off with sim = 0. In these cases the securities market outcomes resemble the unsecured lending policy. As under the unsecured lending policy, if rR < rpen then there is some b at which the interest rate places an upper bound on market illiquidity at rR/(1 + rR). Alternatively, if rR > rpen then condition (ii) never holds.
Lemma 3. For given symmetric s > 0, if rR ≤ ru then expected market illiquidity m* (across b) is strictly lower under the secured lending policy than under the unsecured lending policy, because there is necessarily some b at which the collateral constraint Equation (17) binds.
Figure 4 illustrates Lemma 3. A bank's collateral constraint binds whenever its collateral obligations force it to divert securities away from another use. Collateral constraints necessarily bind for high enough liquidity shocks, when banks have large borrowing requirements and most of their securities are being provided as collateral. If rR is sufficiently high, collateral constraints also bind for smaller shocks, when banks' borrowing needs are low but they would still prefer to sell all their securities and borrow less. Either way, binding collateral constraints mean fewer securities are sold, and market illiquidity is lower, than would be the case without collateral constraints.[11]
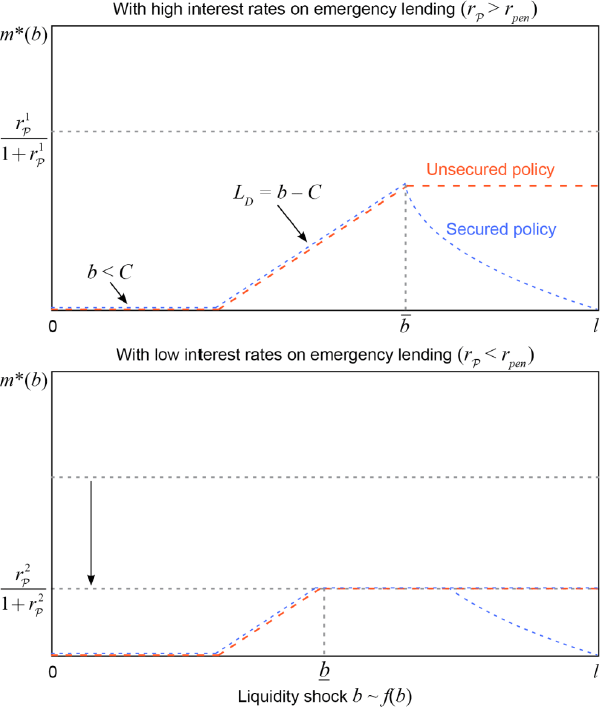
Notes: The two panels illustrate market-clearing market illiquidity m* across b, holding s constant, for two different interest rates , but equal interest rates across the two policies. In the lower panel, , imposes a bound on market illiquidity.
When Equation (17) binds, Equations (10) and (17) hold with equality, implying that bank i's borrowing is
and securities sales are
A binding collateral constraint means that the quantity of securities sold (l – b)/m* does not depend on securities held si, because liquidity deficiency implies that any increase in si (and corresponding reduction in ci) raises bank i's borrowing needs one for one. Therefore, any additional securities held are simply used as collateral. If h were assumed positive, sim would be negatively related to si, because an increase in si would raise borrowing needs by more than the additional securities could collateralise.
Therefore, if , bank i's ex post pay-off (from Equation (9)) is
The return on liquidity rR /m* is the interest cost on necessary borrowing, because collateralising one unit of borrowing means losing 1 – m* liquidity through foregone sales, so to obtain one more unit of liquidity, borrowing must increase by 1/m*. This imposes (1 + rR)/m* repayment cost and saves 1/m* on securities used as collateral rather than sold. Observe that m* = rR /(1 + rR) implies 1/ (1 – m*) = rR / m*, so the ex post pay-off satisfies Equation (20) whenever .
Bank i's pay-off is therefore
Proposition 4. Assuming symmetric choices s, there is a unique equilibrium . If rs is low enough relative to rR is strictly decreasing in rR, bound below only at zero. If the optimal unsecured policy equilibrium is interior, the optimal secured policy equilibrium has higher W.
Market illiquidity negatively affects bank profits, evident in Equation (21). However, it does not negatively affect the marginal return to securities and incentivise liquidity risk-taking, for two reasons. First, banks individually have no impact on the securities price, so a weakening of the collective impact does not factor into their decision-making. Second, banks with more liquidity risk are just as constrained from participating in the securities market than banks with fewer securities, because they need to collateralise more borrowing. This means that the avoided losses from improved market liquidity are not positively related to ex ante securities holdings. The overall result is that the collateral constraint improves market liquidity, offsetting fire sale externalities, and reducing banks' losses without incentivising greater liquidity risk-taking.
The model demonstrates several other features of a secured lending policy. As mentioned earlier in this section, the authority's haircuts need to be set to ensure banks can get more liquidity from their securities than the private market offers them, or otherwise the policy has little capacity to save banks. Allen and Carletti (2008) make a similar recommendation, arguing that collateral should be valued based on what the price would be in a liquid market, using models and inputs such as prices in other markets that are active. They relate this to the Bagehot (1920) recommendation that ‘advances should be made on all good banking securities’ (p 188) and explain that it is permitted by accounting standards. This principle was arguably followed in 2008 and 2009, when many central banks substantially widened the range of repo-eligible collateral, after private markets for those securities dried up. Cecchetti (2009) writes about the Fed's emergency lending:
The rules of the Term Auction Facility allow banks to pledge collateral that might otherwise have little market value. Under the rules of the auctions, TAF loans must be over-collateralized by at least a factor of two, but in reality the Fed is taking collateral at a price that is almost surely above its actual market price (p 67).
A related implication of this collateral valuation principle is that central banks' haircuts during liquidity crises should not be calibrated only on securities price volatility, which is typical practice during normal times and for haircuts in private markets. If securities price movements reflect market illiquidity, they do not necessarily imply riskier collateral for the central bank, which is not susceptible to liquidity shortages. More importantly, the principle demonstrates that unnecessarily high haircuts can lead to illiquidity-driven bank failures, having the perverse effect of increasing the central bank's counterparty risk rather than decreasing it.
Another policy feature illustrated by the model is that the collateral constraint, when binding, forces banks to borrow more than they would without a constraint, because every unit of borrowing means 1 – m* less liquidity that can be drawn from the securities market. Relative to an unsecured lending policy, this amplifies the penalising effect of a given level of penalty rates, by increasing the borrowing that takes place at those rates. Corollary 5 states this result.[12] Holding liquidity risk-taking constant, this also implies more expansion of the authority's balance sheet for a given shock. A cost of the balance sheet expansion is that a bank's other creditors lose any claim on securities the bank provides the authority as collateral, if the bank were to default at date 2. However, this section has shown that the authority's trade-off between liquidity risk and banking system profits is weaker under the secured policy, so the authority may opt for greater penalties and therefore less borrowing, relative to an unsecured lending policy. Further, the authority arguably takes on less balance sheet risk under the secured lending policy, because its lending is collateralised.
Corollary 5. If rR = ru > rpen then whenever S* < l.
5. Liquidity Support through Securities Purchases
Under a securities purchase policy, the authority ensures that the liquidity value of banks' portfolios remains sufficiently high to avoid failure. It does this by purchasing securities to lift their market-clearing price. The policy parameter is a purchase schedule mS(b) such that if without intervention market illiquidity m* would be above mS(b), the authority purchases enough securities to ensure the market price is 1–mS(b).
A particular purchase schedule can be represented by the level of such that any bank with is saved by the policy. Specifically, provided that no bank fails,
and the authority intervenes at all b > bS such that
However, the authority's priority of preventing bank failures implies that if for any i, it will respond to banks' choices by raising to maxi{si}. The date 1 realisations of bS and can be written and to incorporate this requirement. Bank i's pay-off is
Proposition 6. Say an unsecured lending policy with ru > rpen induces an equilibrium . Under a securities purchase policy, the authority cannot credibly induce an equilibrium at or below .
Proposition 6 is a result of banks understanding that the authority will not let them fail, which permits each bank to lower by choosing . The preceding analysis has been intentionally agnostic about the functional form of the cost of failure to banks, but the result would be the same for any positive cost, because banks know the authority will not let them face these costs. Moreover, banks benefit from raising , because it lifts the price that the authority offers in the case that . Therefore, banks have incentives to raise their liquidity risk-taking to levels of that are credible.
Proposition 6 does not require that the authority is unwilling to let any banks fail. Consider an alternative objective function W in which = 1 if and only if some particular positive measure of banks fails, and assume the cost of failure to banks is sufficiently high that banks always choose to avoid it. This would produce multiple equilibria, with one at and one at higher S.[13] Only the highest equilibrium is time consistent for the authority, because the authority will concede to liquidity risk levels above provided that the sufficient measure of banks coordinates above it. In other words, individual banks' liquidity risk choices are strategic complements, as in the model of Farhi and Tirole (2012).
This need to be more lenient to banks when they take more liquidity risk, and the consequential lack of credible deterrence, occurs because the policy does not require banks to commit future assets. The authority only receives compensation (i.e. securities) for its liquidity provision at the height of the crisis, when banks have little to compensate without failing. The lending policies, on the other hand, do not require payment from banks until conditions improve. The model differs from that of Farhi and Tirole (2012) by having a disconnect between the market's date 1 provision of liquidity and the date 2 value of banks' assets. This disconnect presents an opportunity for a liquidity-rich authority. It can require date 2 repayments that are large enough to deter ex ante liquidity risk-taking, without undermining its primary objective of preventing bank failures during the peak of the crisis.
6. Model Generalisations and Extensions
One of the simplifying model features maintained in previous sections is that there are no interbank transactions in equilibrium. Interbank transactions are not ruled out by the model structure, but do not occur in symmetric equilibria because the liquidity shock is homogeneous. This is not important for the results. To generate asymmetry in ex ante securities holdings, some banks could expect securities to pay zero net returns in the no-shock outcome, i.e. rs = 0. These banks would hold only cash at date 0, but at date 1 profitably purchase securities from other banks if m* > 0. It is straightforward to show that, for the other banks, the previous results are unchanged – the effect is similar to an upward pivot of LS, which is nested in the baseline model. Banks' liquidity positions affect each other only through the date 1 market return on liquidity, without implications for how distressed banks deal with the authority, aside from potentially changing the amount of intervention required.
In the baseline model, the impact of the shock is endogenous to banks' choices, but the shock itself is not. It is feasible that creditors might withdraw more at date 1 from a bank that has taken more liquidity risk at date 0. This could be incorporated by assuming that the liquidity shock is drawn from f (b, si), where implies stochastically dominates . This adds terms to the marginal return on securities that make existence proofs difficult. However, there is little reason to expect conclusions about the differences between liquidity injection policies to change. The generalisation is likely to simply lower the incentives for liquidity risk-taking under the lending policies, without direct implications for how collateral constraints offset fire sale externalities.
6.1 Capital Injection Policies and Policy Combinations
To generate insights about a capital injection policy, the model can be examined from an individiual bank's perspective, holding the behaviour of other banks constant. The policy involves the authority providing banks liquidity in return for a stake in their equity. To incorporate equity into the model, at date 0 each bank is endowed with long-term non-marketable assets that cannot be sold at date 1, and that have positive value at date 2. Denote the date 2 value as ai, which may vary across i. At date 1 the authority provides bank i liquidity in return for a share of bank i's date 2 value, where is bound below one.
Assume that the authority only provides bank i liquidity that it cannot raise itself. This removes emphasis from the specific functional form of , because banks do not try to balance the marginal costs across date 1 liquidity sources. It contrasts with the lending policies in Sections 3 and 4, into which banks self-select. Indeed, in reality capital injections have often been targeted at specific banks, whereas lending policies have been offered on a broader basis, through auctions, standing facilities, or voluntary take-up of government guarantees (see Appendix A for examples).
Under the capital injection policy C, bank i's pay-off is
Proposition 7. Under the capital injection policy, any interior equilibrium is characterised by liquidity risk si being weakly decreasing in long-term assets ai.
Corollary 8. The capital injection policy cannot leave a bank with a negative date 2 pay-off whereas lending policies can.
Proposition 7 and Corollary 8 reflect the same intuition – when the authority buys a stake in banks' equity, banks with low equity have little to repay. The policy is unable to disincentivise their liquidity risk-taking, and, despite being close to insolvency, does not leave them insolvent ex post. Avoiding ex post insolvency has costs and benefits. Work on gambling for resurrection argues that low-profitability banks tend to take excessive risks (e.g. Freixas and Rochet 2008, chapter 9). On the other hand, if external creditors' behaviour is endogenous to banks' ex post pay-offs, heightened concerns about ex post solvency could amplify the date 1 liquidity distress (e.g. Rochet and Vives 2004; Morris and Shin 2016). In this case, by Corollary 8, a capital injection may help to stave off further runs. He and Krishnamurthy (2013) also argue that a capital injection can improve banks' access to credit.
To acknowledge this potential benefit, the model could be generalised to permit the authority to combine the capital injection policy with either an unsecured or secured lending policy. If banks are allowed to choose the proportion of their liquidity deficit that is funded by the capital injection, an immediate implication is that no banks would be insolvent at date 2. That is, banks' date 2 profit is positive if they fund the deficit completely through the capital injection (Corollary 8), so date 2 pay-off under their optimal choice must be at least as high. The policy combination would preserve the benefits of secured lending over unsecured lending if collateral constraints still bind for high liquidity shocks. If this would not occur with h = 0, it could be induced with h > 0. Formal treatment is left for future work.
6.2 Penalty Rates and Heterogeneous Securities
The conclusions in this paper relate to lending policies that charge penalty rates, a topic with a small literature of its own. Bagehot (1920) highlights the benefit of deterring excessive expansion of the authority's balance sheet; Fischer (1999) explains that penalty rates disincentivise liquidity risk-taking; further reading includes Freixas, Rochet and Parigi (2004), Rochet and Vives (2004) and Castiglionesi and Wagner (2012). As Rochet and Vives (2004) demonstrate, penalty rates can have the undesired effect of discouraging private creditors by lowering banks' expected future profits. This topic is briefly discussed in Section 6.1.
Following Bagehot (1920), penalty rates in this paper are defined relative to outside funding options; that is, relative to securities market liquidity. It is interesting to think about the case in which rP is penalising relative to some securities but not others. Consider a heterogeneous range of securities ranked by liquidity risk and indexed by j, such that j = 1, …, J. Specifically, for equal LD > 0 in each securities market, market illiquidity is ranked . Fix b, s and the lending policy interest rate rP, defining mP such that rP = mP/(1 –mp) and 0 < mP < 1. For securities with , rates are penalising and banks prefer selling them over emergency borrowing. For securities with , banks prefer borrowing from the authority, which binds these securities' market illiquidity above at mP.
Assume a bank is liquidity deficient, so it cannot raise enough liquidity by selling all its securities. Under an unsecured lending policy, it sells all its securities with . It is indifferent between selling securities with and borrowing, so its borrowing ei is somewhere between its remaining liquidity needs when selling all these securities and when selling none of them. Under a secured lending policy it must provide collateral to the authority. It starts by providing securities with , but if these are not sufficient, additionally provides securities whose sales generate the largest losses. In this case there is some for which it sells all securities with and provides the rest as collateral. The implication is that, relative to an unsecured lending policy, the collateral constraints reduce market illiquidity for the most illiquid securities that are accepted as collateral.
7. Conclusion
This paper models a liquidity crisis in the banking system and compares different types of emergency liquidity injections. A crisis occurs when there is an exogenous system-wide withdrawal of funding liquidity that forces banks to sell securities, causing securities price depression to the point that banks cannot survive the withdrawal. The banking system's level of pre-crisis exposures to securities with liquidity risk determines (inversely) how large the outflow must be to cause bank failures, and hence the likelihood of a crisis occurring.
A secured lending policy can mitigate banks' fire selling of securities, because banks must use those securities as collateral for borrowing from the authority. The positive effect of the constraint essentially offsets banks' fire sale externalities – neither are factored into banks' profit maximisation – and so does not incentivise liquidity risk-taking. Accordingly, relative to an unsecured lending policy, a secured lending policy can reduce banks' losses on illiquid securities without greater incentives for liquidity risk-taking. Under either lending policy, banks' incentive to take liquidity risk is decreasing in the interest rates on emergency lending. For penalising interest rates to not cause further bank failures, however, lending must be for long enough terms that the liquidity distress has subsided before repayments are due. Liquidity injections via asset purchases involve no ex post repayments, and therefore, relative to lending policies, have less ability to save banks and disincentivise liquidity risk-taking.
The model presented here is general in several dimensions that could be restricted for applications in more complex settings. There is a continuum of banks and an authority that all react optimally to each other, with continuous liquidity shocks and investment choices, and endogenous market liquidity and crisis probability. By simplifying aspects of the model, such as discretising variables or agents, it could be applied to a wide variety of other settings. For example, the endogenous crisis probability may be suitable for analysing financial cycles. This is left to future work.
Appendix A: Emergency Liquidity Injection Policies in Europe and the United States
This appendix reviews some of the largest liquidity injection policies adopted by authorities in Europe and the United States around late 2008. Both regions utilised secured lending, bank equity purchases, and unsecured lending subsidisation. Unsecured lending subsidisation was mostly through government guarantees on banks' unsecured debt, with also some direct loans to banks. The United States relied most heavily on secured lending; in Europe, government guarantees on banks' unsecured debt were more prominent.
A.1 Europe
The ECB increased secured lending by modifying and expanding its existing tools for open market operations. These were the main refinancing operations (MRO) and longer-term refinancing operations (LTRO), under which banks borrowed from the ECB against eligible collateral. MROs were previously conducted weekly with one-week maturity and LTROs typically conducted monthly with maturities of one month and longer. In March 2008, the ECB announced it would run a series of LTROs with six-month maturities, compared to maturities of three months in previous LTROs. Prior to October 2008, the ECB priced the MROs and LTROs by taking bids from banks comprising interest rates and corresponding quantities, auctioning a predetermined total amount at the lowest successful interest rate bid; the amount auctioned was calibrated to leave the outcome interest rate a certain level above the ‘deposit rate’ that the ECB pays banks on their overnight cash holdings. On 15 October for MROs and 30 October for LTROs, the ECB switched to a fixed rate tender with full allotment, whereby it fixed the interest rate and banks could borrow any amount requested. On 23 October, the ECB also substantially widened the range of eligible collateral, accepting more corporate debt instruments and lowering the required credit rating of collateral from A– to BBB–. The most rapid and substantial increase in MRO and LTRO lending occurred between late September and late October 2008, rising from €480 billion to €820 billion. Over the same period the interest rate on the ECB's lending declined from around 4.7 to 3.75 per cent, above the interbank rate (EONIA) which declined from 3.70 to 3.55 per cent.
In mid October, several European countries offered government guarantees on banks' newly issued debt, guaranteeing around €770 billion, and often charging prices estimated to be close to market rates in normal times.[14] Around the same time, some of these countries engaged in bank recapitalisation schemes, with a combined cap of €140 billion, and purchased or guaranteed around €43 billion of banks' assets.[15] A large component of this was the Swiss National Bank's (SNB) transaction with UBS, under which the SNB created a ‘bad bank’ fund, owned and mostly funded by the SNB, that purchased around €30 billion worth of assets from UBS with an arrangement that the SNB would receive UBS shares if the bad bank eventually made a loss. The transaction therefore had similarities to an equity purchase. In November 2013, the SNB sold the last of the fund back to UBS and announced that it made around €2.8 billion capital gains on top of interest payments.
A.2 The United States
Throughout the crisis the US Federal Reserve introduced a number of new facilities for collateralised open market operations, including the term auction facility (TAF), the primary dealer credit facility (PDCF) and the term securities lending facility (TSLF).[16] The TAF, introduced in March 2007, lent to a wide range of depository institutions – in contrast to the standard open market operations that only transact with the 20 or so primary dealers – for terms of one or three months, via single price auctions each of a fixed total amount. The largest monthly increase in TAF lending was from US$125 to 390 billion between early October and early November 2008. The PDCF, introduced in March 2008, lent funds without limit to primary dealers on an overnight basis, at the Fed's overnight policy rate and with an additional frequency-based fee for each loan to a borrower that had used the facility more than 45 times. The TSLF, also introduced in March 2008, made one-month loans of Treasury securities to primary dealers, collateralised by other securities, through single price auctions. In mid September 2008, the Fed widened its acceptable collateral for the PDCF – from investment-grade securities to those typically accepted in private repo markets – and the TSLF – from certain types of AAA securities to any investment-grade debt instruments. From mid September to early October 2008, TSLF loans outstanding rose from US$135 billion to 275 billion, and overnight lending under the PDCF rose from zero to US$155 billion.
US authorities also injected substantial liquidity using unsecured debt subsidisation and bank equity purchases. On 7 October 2008, the Fed introduced the commercial paper funding facility (CPFF) to purchase newly issued commercial paper of three-month maturity from a wide variety of companies, with a substantial proportion from the banking sector. It purchased unsecured commercial paper (essentially making unsecured loans), charging the overnight index swap (OIS) rate plus 100 basis points, and asset-backed commercial paper (ABCP), charging the OIS rate plus 300 basis points. By the end of October the Fed owned US$157 billion of unsecured commercial paper and US$94 billion of ABCP. On 14 October 2008, the FDIC implemented the temporary liquidity guarantee program (TLGP), which guaranteed without limit banks' newly issued unsecured debt, charging a 75 basis point fee for any loan that applied the guarantee. TLGP-guaranteed debt outstanding reached US$224 billion by the end of 2008, later peaking at US$336 billion.
Bank equity purchases in the United States were made under the Troubled Assets Relief Program (TARP). The largest part of TARP was the Capital Purchase Program (CPP), through which on 28 October 2008 the US Treasury purchased US$115 billion of equity and warrants from 8 of the largest US banks, and by February 2009 had disbursed a total of US$194 billion to 317 different financial entities. Additional TARP funds were spent on institution-specific purchases, providing AIG US$40 billion on 10 November 2008 – which it used to partially repay a senior unsecured loan of US$85 billion from the Fed made on 15 September – and providing Bank of America US$20 billion on 16 January 2009. The US Treasury made positive returns on the CPP and both institution-specific purchases.
Appendix B: Proofs
Definition. Throughout these proofs, refer to generalised derivatives, defined, as in Clarke (1975), as the convex hull of the set of limits of the form df (x + hi)/dx and where . In any neighbourhood such that f is continuously differentiable, the generalised derivative collapses to the standard derivative.
Remark. In most cases throughout these proofs, is a function of the almost everywhere continuously differentiable functions m* and . In such cases the generalised derivatives of with respect to si or S are equal to the interval in between the left-hand and right-hand derivatives
Lemma 9. Under the unsecured lending policy, market illiquidity m* is Lipschitz continuous, almost everywhere differentiable, and non-decreasing in b, and, given s−i = S, in S.
Proof. Market illiquidity m* is defined implicitly by gm = 0 where
If defined in Equation (13), then LD is whatever value that makes m* constant at its upper bound ru /(1 + ru), by the arbitrage condition discussed in Section 3. The rest of this proof fixes ru and considers . In these cases LD is defined by
where
If then, in aggregate, banks have sufficient cash to meet the liquidity shock, so no securities are sold to securities buyers and m* = 0. Market illiquidity m* continuously increases in b from zero as banks' aggregate cash shortage LD = b + S – l continuously increases in b through zero, by the properties of LS. At positive m*, bank i can be liquidity deficient such that Li = si (1 – m) as in Equation (B3). The next paragraph considers positive m*.
First assume (almost-) symmetric s, i.e. s−i = S. If S is high enough then there is some such that the unit measure of banks is just liquidity deficient, and M (b' + S – l) = (l – b') / S. (If S is not high enough, then LD = b + S – l for all and the following arguments hold more trivially.) At this b' liquidity demand is LD = b + S – l = S(1 – m), so LD defined by Equations (B2) and (B3) is continuous in a neighbourhood of b. The left-hand derivative is d–LD/db = 1, and the right-hand derivative is d+LD/db = 0, which are both bounded, so LD is Lipschitz continuous. Further, given that , the function gm is strictly increasing in m whether LD = l + b – S or LD = S(1 – m). Therefore gm satisfies the Lipschitz implicit function theorem and m* is Lipschitz continuous.
Total differentiation of gm at gm = 0 shows that have the same signs as , so, from Equations (B2) and (B3), m* is non-decreasing in b and S.
Observe that LD is continuously differentiable at all b such that , from Equations (B2) and (B3). Therefore, by the implicit function theorem, when s–i = S, market illiquidity m* is almost everywhere continuously differentiable.
Now consider asymmetric s. It is possible that for some , only a proportion of banks are liquidity deficient. Banks' aggregate liquidation can therefore be expressed as , where , and S1 and S2 are the mean securities holdings for banks with respectively. For any (m, b) such that a positive measure of banks holds si = (l – b)/m, the proportion of liquidity-deficient banks is discontinuous. Nevertheless, si = (l – b)/m implies b + si – l = si(1 – m), so LD is still continuous in all variables. To see Lipschitz continuity and the derivative signs for LD, the same reasoning as for the symmetric case can be applied to the points of non-differentiability. It follows that if then, in general, gm is Lipschitz continuous, strictly increasing in m, and non-decreasing in b, so m* is Lipschitz continuous and non-decreasing in b. Because there is a finite measure of banks, the set of b such that is discontinuous has measure zero, so m* is almost everywhere continuously differentiable.
Lemma 10. Under the unsecured lending policy, for all , the liquidity deficiency threshold is Lipschitz continuous, almost everywhere differentiable, and strictly decreasing in si, and, if s–i = S, also in S.
Proof. The liquidity deficiency threshold is defined implicitly by gb = 0 where
Lemma 9 shows that m* is non-decreasing, Lipschitz continuous, and almost everywhere continuously differentiable in b and (when s–i = S) in S. It follows that gb is strictly decreasing in b. Therefore, by Equation (B4) and implicit function theorems, is Lipschitz continuous and almost everywhere continuously differentiable in b and S.
From Equation (B4) gb is clearly continuously differentiable in si. Total differentiation of gb shows that
Lemma 11. Assume , where is an arbitrarily small constant. References to S assume s–i = S. Under the secured lending policy, market illiquidity m* is Lipschitz continuous and almost everywhere differentiable in b and in S, and non-decreasing in S.
Proof. Under the secured lending policy each bank's securities liquidation satisfies
Most of the reasoning in the proof of Lemma 9 also applies here (excluding proof that m* is non-decreasing in b, which is not part of this Lemma). The only two points of departure are that: (i) while is bounded for the unsecured lending policy, it remains to be shown for the secured lending policy, due to the difference between Equations (B3) and (B5); and (ii) given that m* is not necessarily non-decreasing in b, it remains to be shown that the set of b such that m* is not differentiable has measure zero.
- If b > l – sim then dLi/db = 1 – 1/m. As b approaches l – sim from above, this derivative approaches 1 – si/(l – b). It follows from that , and therefore is bounded.
-
Lipschitz continuity of LD is given by (i). Since Li and therefore LD is non-increasing in m, LS – LD is strictly increasing in m, so the Lipschitz implicit function theorem applies to define m*. Then, by the (standard) implicit function theorem, m* is continuously differentiable in any (b, S) such that LD is continuously differentiable. As in the proof of Lemma 9, non-differentiable points of LD can only occur at (b, S) such that a positive measure of banks is on the threshold of liquidity deficiency. These points must therefore satisfy b = l – sim*(b, S) where si equals some s0 such that a positive measure of banks holds si = s0. The following shows that the measure of such (b, S) is zero.
Say there is a non-degenerate interval of b or S such that b = l – s0m*(b, S). Inside the bounds of this interval, the proportion of liquidity-deficient banks is constant, so , which is continuously differentiable. Therefore non-differentiable points in LD are limited to the boundary points of such intervals. Further, there is a finite measure of banks, so the set of si satisfying the definition of s0 has measure zero, and the number of such intervals is countable. Therefore the set of non-differentiable points of LD has measure zero.
Remark. Under the secured lending policy, as b → l, banks' ability to handle market illiquidity diminishes to zero. Therefore their securities selling also diminishes to zero, as they instead use all their securities as collateral to borrow from the authority. For simplicity the model has assumed all market illiquidity is generated by banks, so b → l then implies m* → 0, and at the limit some of the reasoning in the proof of Lemma 11 breaks down (i.e. is not bounded). This is clearly an unrealistic outcome – for the highest possible liquidity shocks, securities markets approach full liquidity – so it is assumed away for Lemma 11, because it has an arbitrarily small probability. This could be justified by an assumption that while banks believe that .
Alternatively, the situation can be ruled out by assuming that whenever there is a liquidity shock, there is also exogenous liquidation in the securities market, where is arbitrarily small, and rs is above some lower bound (which compensates banks for the increased liquidity risk from ). All the results in this paper would be maintained under this assumption.
Lemma 12. Under the secured lending policy, for all , the liquidity constraint is Lipschitz continuous, almost everywhere differentiable, and strictly decreasing in si, and, if s–i = S, weakly decreasing in S.
Proof. The liquidity constraint is defined by gb = 0, where gb has the same form as in Equation (B4). The proof of Lemma 10 also applies here, once it is shown that
which is required for applying the Lipschitz function theorem to show that is unique given (si, s–i). Re-expressing the LD function allows us to evaluate this derivative. Fix s, and denote the total securities held by banks with i ≤ x as Fs(x), remembering that si is non-decreasing in i (see Section 2.1). Define such that and . This permits the expressions for LD and Li to be combined into
The proof of Lemma 11 shows that LD is almost everywhere continuously differentiable, with any non-differentiable points aligning with non-differentiable points of m*. Therefore the left-hand and right-hand derivatives of LD are defined for all b. To solve them, first observe that, where dm*/db is defined,
Then, using Equation (B4), and acknowledging that gb = 0 implies m* > 0,
Positivity of this derivative means that the Lipschitz implicit function theorem applies, and is unique and Lipschitz continuous. Further, discontinuities in the derivatives of gb occur only at discontinuities in the derivative of m*, so is almost everywhere continuously differentiable by the implicit function theorem. The signs of the derivatives of then follow from total differentiation of gb.
Proof of Proposition 1. This proof will show that: (i) is continuous; (ii) is non-positive; (iii) is negative; and (iv) if S > 0 then is negative. From (i), (ii) and (iii) it follows that if , there is a unique fixed point such that . From (iv), this fixed point is continuously and strictly decreasing in ru. Finally, the proof will demonstrate that (v) S* is bound below only at zero.
-
The integrands in Equation (16) are equal at , so
(B9)where and m* are Lipschitz continuous functions by Lemmas 9 and 10. Therefore Equation (B9) is continuous.
-
For high enough si it is the case that and
(B10)Lemmas 9 and 10 show that . Additionally, implies . Therefore implies Equation (B10) is negative. Alternatively, if then, from Equation (B9) and the fact that , it follows that .
-
If then
(B11)Alternatively, if then each in Equation (B11) is replaced by , and the third term is zero because implies 1 + ru = 1/(1–m*). Lemma 9 shows that , and if b is just above l – S, then . Therefore the sum of the first two terms in Equation (B11) is negative. Further, if then 1 + ru > 1/(1–m*), and Lemma 10 shows that , so the third term in Equation (B11) is also negative. Therefore .
-
Whether
(B12)This is true because if then and the two integrands in Equation (B9) are equal at , so the integral-limit terms in the derivative drop out. If S > 0 then at high b, m* > 0, and whenever si > 0 it is the case that , so S > 0 implies that Equation (B12) is negative for all si > 0.
-
If S = 0 then m* = 0 for all b so and . Therefore there can be no equilibrium at S* = 0. However, for any given is less than l and Equation (B9) is decreasing without bound in ru. Therefore, high enough ru can generate negativity of Equation (B9) whenever s > 0.
Proof of Lemma 2. Banks' pay-offs, expressed in Equation (16), are decreasing in market illiquidity m*, which, by Lemma 9, is increasing in S whenever m* > 0. The marginal return to securities in an aggregated pay-off function includes this negative effect, whereas Equation (B9) does not. Therefore, for any given S, the collective marginal return to securities is lower than the individual marginal return, so if the model equilibrium is interior, then the collective equilibrium is lower.
Proof of Lemma 3. This result is illustrated in Figure 4. Fix symmetric s > 0 across both policies. For low b, securities liquidation satisfies LD = b + S – l and market illiquidity satisfies . As b increases, this holds true until m* hits an upper bound, either at rP / (1 + rP) or at M(S(1 – m*)).
At higher is constant, whereas is weakly decreasing and, for some b, strictly decreasing. Specifically, is constant if bound at rR / (1 + rn) and decreasing otherwise. The result then follows from rR ≤ ru and from M(S(1 – m*)) being equal across policies. It only remains to be shown that is strictly decreasing for some b.
Binding collateral constrains are the cause of decreasing . To see that this necessarily occurs, first observe that if , then Equation (17) binds at all (see the discussion in Section 4). If then for to simultaneously satisfy Equation (3) and , the total quantity of securities sales Sm must satisfy such that
Given this, collateral available for borrowing is S – Sm, which is less than the required borrowing if
This condition can be rearranged to where
The properties of LS imply that . Therefore Equation (B13) is necessarily violated for some b.
Proof of Proposition 4. The first two sentences of Proposition 4 have a similar proof to Proposition 1. Only the following changes are required:
- References to u, Equation (16) and Lemmas 9 and 10 are replaced by references to R, Equation (21) and Lemmas 11 and 12, respectively.
- In Equations (B9), (B10) and (B11), the term 1 + ru is replaced by rR /m*(b,s–i). In some cases the m* in this denominator cancels another m* in the expression. For instance, Equation (B9) is replaced by
- In Equation (B12), the –m* term becomes –1.
The following proves the third sentence of Proposition 4. It is shown that if , then, in equilibrium, . This implies that for any interior optimal unsecured policy equilibrium, there is a secured policy equilibrium at the same S* with higher W. Therefore W is maximised at a higher level under the secured policy.
Fix symmetric , which sets constant and equal in both policies, denoted . Note that for , under the unsecured policy m*(b) is constant. Denote this . Denote market illiquidity under the secured lending policy . From Equations (16) and (21), the secured policy has a higher pay-off than the unsecured policy if and only if
Further, equal interior equilibrium S implies . Therefore, from Equations (B9) and (B14),
Substituting Equation (B16) into Equation (B15) and rearranging gives the condition
This condition holds if for all , and with a strict inequality for some positive measure of b. This is true if ru ≥ rR by Lemma 3. In turn, ru ≥ rR is implied by Equation (B16) and the arbitrage condition from Equation (12). That is, combining these two conditions implies that either and ru > rR.
Proof of Corollary 5. Given fixed S and the inequalities rP > rpen and , the ex post (i.e. conditional on b) marginal return to securities under the secured lending policy and under the unsecured lending policy. The condition ru > rpen implies that for all b, which can be rearranged to . Therefore rR = ru implies . So if , under the secured lending policy the marginal return to securities at is negative, and, by concavity of (shown in Proposition 4), must be lower.
Proof of Proposition 6. Without loss of generality set . Under a securities purchase policy the marginal return to securities can take two forms, depending on whether . From Equation (24), if it is
and if it is
Holding (si, s–i) constant, when ru > rpen, which implies , both Equations (B17) and (B18) are greater than Equation (B9). To see this, consider the ex post marginal returns, i.e. Equations (B9), (B17) and (B18) conditional on a specific realisation of b. They are equal across policies for . For higher b, the ex post marginal return necessarily decreases under the unsecured lending policy, by ru > rpen and the fact that m* is increasing in b when positive (Lemma 9). For higher b under the securities purchase policy, however, the ex post marginal return does not decrease, because (i) when is not less than –m*(bS) and is increasing in b at higher b, and (ii) when it is zero. Therefore, in expectation across b, both Equations (B17) and (B18) are greater than Equation (B9).
Now fix the induced by an unsecured lending policy with ru > rpen. Note that is positive for any (si, S) satisfying , by negativity of Equations (B10) and (B11), and because . Therefore, by the reasoning in the previous paragraph, is positive for any (si, S) satisfying , and so at and there can be no equilibrium at .
Proof of Proposition 7. The ex post marginal return to securities for given which, for given s, is strictly decreasing in ai. Therefore, the ex ante marginal return to securities, and any interior optimum, are also strictly decreasing in ai.
Proof of Corollary 8. The result follows directly from Equation (25) the assumption that .
References
Acharya VV, HS Shin and T Yorulmazer (2011), ‘Crisis Resolution and Bank Liquidity’, The Review of Financial Studies, 24(6), pp 2166–2205.
Acharya VV and T Yorulmazer (2008), ‘Cash-in-the-Market Pricing and Optimal Resolution of Bank Failures’, The Review of Financial Studies, 21(6), pp 2705–2742.
Allen F and E Carletti (2008), ‘Mark-to-Market Accounting and Liquidity Pricing’, Journal of Accounting and Economics, 45(2–3), pp 358–378.
Allen F and D Gale (1994), ‘Limited Market Participation and Volatility of Asset Prices’, The American Economic Review, 84(4), pp 933–955.
Ashcraft A, N Gârleanu and LH Pedersen (2011), ‘Two Monetary Tools: Interest Rates and Haircuts’, in D Acemoglu and M Woodford (eds), NBER Macroeconomics Annual 2010, 25, University of Chicago Press, Chicago, pp 143–180.
Attinasi MG (2010), ‘Euro Area Fiscal Policies: Response to the Financial Crisis’, in A van Riet (ed), ‘Euro Area Fiscal Policies and the Crisis’, European Central Bank Occasional Paper Series No 109, pp 12–21.
Bagehot W (1920), Lombard Street: A Description of the Money Market, New edn with an Introduction by H Withers, E.P. Dutton and Company, New York.
Basel Committee for Banking Supervision (2013), Basel III: The Liquidity Coverage Ratio and Liquidity Risk Monitoring Tools, Bank for International Settlements, Basel.
Bernanke BS (2008), ‘Liquidity Provision by the Federal Reserve’, Speech given at the Federal Reserve Bank of Atlanta Financial Markets Conference ‘Financial Market Reforms: Taking Stock’, Sea Island, 12–14 May.
Brunnermeier MK (2009), ‘Deciphering the Liquidity and Credit Crunch 2007–2008’, The Journal of Economic Perspectives, 23(1), pp 77–100.
Brunnermeier MK and LH Pedersen (2009), ‘Market Liquidity and Funding Liquidity’, The Review of Financial Studies, 22(6), pp 2201–2238.
Cassola N and F Koulischer (2019), ‘The Collateral Channel of Open Market Operations’, Journal of Financial Stability, 41, pp 73–90.
Castiglionesi F and W Wagner (2012), ‘Turning Bagehot on His Head: Lending at Penalty Rates When Banks Can Become Insolvent’, Journal of Money, Credit and Banking, 44(1), pp 201–219.
Cecchetti SG (2009), ‘Crisis and Responses: The Federal Reserve in the Early Stages of the Financial Crisis’, The Journal of Economic Perspectives, 23(1), pp 51–75.
Chernenko S and A Sunderam (forthcoming), ‘Do Fire Sales Create Externalities?’, Journal of Financial Economics.
Choi DB, JAC Santos and T Yorulmazer (2019), ‘A Theory of Collateral for the Lender of Last Resort’, Unpublished manuscript, January. Available at <https://dx.doi.org/10.2139/ssrn.2874999>.
Clarke FH (1975), ‘Generalized Gradients and Applications’, Transactions of the American Mathematical Society, 205, pp 247–262.
Coval J and E Stafford (2007), ‘Asset Fire Sales (and Purchases) in Equity Markets’, Journal of Financial Economics, 86(2), pp 479–512.
Diamond DW and PH Dybvig (1983), ‘Bank Runs, Deposit Insurance, and Liquidity’, Journal of Political Economy, 91(3), pp 401–419.
Diamond DW and RG Rajan (2011), ‘Fear of Fire Sales, Illiquidity Seeking, and Credit Freezes’, The Quarterly Journal of Economics, 126(2), pp 557–591.
Farhi E and J Tirole (2012), ‘Collective Moral Hazard, Maturity Mismatch, and Systemic Bailouts’, The American Economic Review, 102(1), pp 60–93.
Fischer S (1999), ‘On the Need for an International Lender of Last Resort’, The Journal of Economic Perspectives, 13(4), pp 85–104.
Fontaine J-S and R Garcia (2012), ‘Bond Liquidity Premia’, The Review of Financial Studies, 25(4), pp 1207–1254.
Freixas X and J-C Rochet (2008), Microeconomics of Banking, 2nd edn, The MIT Press, Cambridge.
Freixas X, J-C Rochet and BM Parigi (2004), ‘The Lender of Last Resort: A Twenty-First Century Approach’, Journal of the European Economic Association, 2(6), pp 1085–1115.
Goldstein I and A Pauzner (2005), ‘Demand-Deposit Contracts and the Probability of Bank Runs’, The Journal of Finance, 60(3), pp 1293–1327.
Gorton G and A Metrick (2012), ‘Securitized Banking and the Run on Repo’, The Journal of Financial Economics, 104(3), pp 425–451.
Hameed A, W Kang and S Viswanathan (2010), ‘Stock Market Declines and Liquidity’, The Journal of Finance, 65(1), pp 257–293.
Hanson SG, A Shleifer, JC Stein and RW Vishny (2015), ‘Banks as Patient Fixed-Income Investors’, Journal of Financial Economics, 117(3), pp 449–469.
He Z and A Krishnamurthy (2013), ‘Intermediary Asset Pricing’, The American Economic Review, 103(2), pp 732–770.
Hordähl P and MR King (2008), ‘Developments in Repo Markets during the Financial Turmoil’, BIS Quarterly Review, December, pp 37–53.
Longstaff FA (2010), ‘The Subprime Credit Crisis and Contagion in Financial Markets’, Journal of Financial Economics, 97(3), pp 436–450.
Merrill CB, T Nadauld, RM Stulz and SM Sherlund (2014), ‘Were There Fire Sales in the RMBS Market?’, Charles A. Dice Center for Research in Financial Economics Working Paper 2014-09.
Morris S and HS Shin (2016), ‘Illiquidity Component of Credit Risk – The 2015 Lawrence R. Klein Lecture’, International Economic Review, 57(4), pp 1135–1148.
Nyborg KG (2017), ‘Central Bank Collateral Frameworks’, Journal of Banking & Finance, 76, pp 198–214.
Nyborg KG and P Östberg (2014), ‘Money and Liquidity in Financial Markets’, Journal of Financial Economics, 112(1), pp 30–52.
Panetta F, T Faeh, G Grande, C Ho, M King, A Levy, FM Signoretti, M Taboga and A Zaghini (2009), An Assessment of Financial Sector Rescue Programmes, BIS Papers No 48, Bank for International Settlements, Basel.
Philippon T and P Schnabl (2013), ‘Efficient Recapitalization’, Journal of Finance, 68(1), pp 1–42.
Rochet J-C and X Vives (2004), ‘Coordination Failures and the Lender of Last Resort: Was Bagehot Right after All?’, Journal of the European Economic Association, 2(6), pp 1116–1147.
Saunders A and MM Cornett (2008), Financial Institutions Management: A Risk Management Approach, 6th edn, McGraw-Hill/Irwin Series in Finance, Insurance and Real Estate, McGraw-Hill/Irwin, New York.
Stein JC (2012), ‘Monetary Policy as Financial Stability Regulation’, The Quarterly Journal of Economics, 127(1), pp 57–95.
Swiss National Bank (2013), ‘UBS Purchases StabFund from the SNB’, Press release, 8 November.
Tirole J (2012), ‘Overcoming Adverse Selection: How Public Intervention Can Restore Market Functioning’, The American Economic Review, 102(1), pp 29–59.
Acknowledgements
The views presented here do not necessarily represent those of the Reserve Bank of Australia. Any errors are my own. For help with this work I owe thanks to Xavier Freixas, Anthony Brassil, Christian Castro, Luci Ellis, Falko Fecht, Fabrizio Germano, Jonathan Kearns, Kebin Ma, Frederic Malherbe, José-Luis Peydró, John Simon and Tomas Williams, among others.
Footnotes
Appendix A provides examples of the various policies. [1]
Unsecured lending policies commonly take the form of government guarantees on banks' unsecured debt issuance, for a pro rata fee that in this paper is interpreted as an interest rate premium. With this type of policy the authority essentially experiences an increase in off-balance sheet contingent liabilities. Still, the authority's exposure to borrowing banks is essentially the same as if it lent directly to banks itself. [2]
The model also illustrates that no matter how loose the haircuts, the authority's counterparty risk is more collateralised than a policy of guaranteeing banks' unsecured debt. [3]
It is a simplifying assumption that, given a liquidity shock, the date 2 securities price is 1 rather than 1 + rs. This could easily be relaxed but would complicate the notation. [4]
[t]he objective of the LCR is to promote the short-term resilience of the liquidity risk profile of banks. It does this by ensuring that banks have an adequate stock of unencumbered high-quality liquid assets (HQLA) that can be converted easily and immediately in private markets into cash … (Basel Committee for Banking Supervision 2013, paragraph 1)The approach also matches Saunders and Cornett (2008)'s textbook definition of liability-side liquidity risk management:
When liability holders demand cash by withdrawing deposits, the [financial institution] FI needs to borrow additional funds or sell assets to meet the withdrawal. The most liquid asset is cash; FIs use this asset to pay claim holders who seek to withdraw funds. However, FIs tend to minimize their holdings of cash reserves as assets because those reserves pay no interest. To generate interest revenues, most FIs invest in less liquid and/or longer-maturity assets. While most assets can be turned into cash eventually, for some assets this can be done only at a high cost when the asset must be liquidated immediately. The price the asset holder must accept for immediate sale may be far less than it would receive with a longer horizon over which to negotiate a sale. (pp 493–494)[5]
A sufficiently high cost of failure would induce the same results. For example, banks could be assumed to hold non-marketable assets with very high liquidation or transfer-of-ownership costs. [6]
The model abstracts from parts of a bank's balance sheet that are not directly relevant to the liquidity dynamics being examined. Accordingly, the fact that the liquidity shock b reduces a bank's asset value, as well as its funding liquidity, is of little concern. This could simply be offset by returning b to bank i at date 2. [7]
This assumes all banks have access to the liquidity injection policy. If not, market illiquidity could be pushed above ru /(1 + ru) by banks without access. In this case the banks with access would not sell any securities and would rely completely on borrowing from the authority. [8]
The condition also rules out asymmetric equilibria by making banks' pay-offs strictly concave. [9]
If h were positive, ei in Equation (17) would be replaced by ei /(1 − h). [10]
The same reasoning implies that the degree of market illiquidity would tend to be decreasing in h. [11]
Higher haircuts would increase the degree of amplification, by requiring banks to divert more securities from selling for a given quantity of borrowing. [12]
Continuity of dsi (Equations (B17) and (B18)) in bs and S ensures existence of the higher equilibrium, potentially at S = l. [13]
The €770 billion figure multiplies 5.7 per cent of euro area GDP (provided in Table 2 of Attinasi (2010)) by 2009 euro area GDP of €12.9 trillion. It excludes guarantees placed in 2009. [14]
These figures are from Attinasi (2010) and Panetta et al (2009). [15]
Some facilities not discussed are: the term asset-backed securities loan facility (TALF) and the large-scale asset purchases (LSAP), aimed at stimulating lending to borrowers outside the financial system; and the money market investor funding facility (MMIFF), targeted at liquidity problems in the money market fund sector. [16]