RDP 9607: Towards an Understanding of Australia's Co-Movement with Foreign Business Cycles 4. The Impact of Foreign Share Markets on Australian Activity

Nicolas de Roos and Bill Russell

November 1996

We now turn to the second area of investigation, that of the share market. The increasing integration of world financial markets allows the rapid propagation of foreign financial prices to domestic financial prices. If capital markets are imperfect, as many authors argue, these shocks to financial prices may lead to changes in real output.[21] Australia's share market is highly correlated with foreign share markets. Table 5a shows the correlation between the quarterly percentage change in the Australian share price accumulation index and similar indexes for the US, Japan, Europe, Australia's export markets, and the ‘world’. McNelis (1993) reports similarly high correlation coefficients for volatility measures of share price indexes for a number of countries. His results are reported in Table 5b. Using Granger causality tests within a VAR model of share price volatilities, McNelis (1993) also shows that the Australian share price index does not help predict a number of the major foreign indexes (reproduced in Table 6). In contrast the share indexes of Germany, Japan, Singapore, UK and the US all ‘Granger cause’ movements in the Australian index. In particular, McNelis identifies the UK as the ‘most significant’ index for predicting the Australian index.

Table 5a: Correlation Between Australian and Foreign Nominal Share Market Returns
  US Japan Europe Export markets World
Correlation 0.62 0.42 0.65 0.61 0.69

Notes: Correlation between the quarterly percentage change in the share market accumulation indexes. Export markets index is calculated as the export weighted average of the accumulation indexes for Japan, US, NZ, South Korea, UK, Singapore, Taiwan and Hong Kong.
Table 5b: Correlation Coefficients of Australian and Foreign Stock-Price Volatility Measures
  Germany Japan Singapore US UK
Correlation 0.31 0.27 0.58 0.68 0.54

Notes: Correlation coefficients of the Schwert Indexes of volatility are reproduced from Panel A of Table 1 in McNelis (1993). Data are end month share price indexes for the period January 1982 to March 1992. Schwert (1988) measures stock market volatility as follows. A 12th order autoregression is estimated for average monthly returns using seasonal dummies. A 12th order autoregression is then estimated for the absolute values of the errors again using seasonal dummies. The predicted values from this regression provide an estimate of the conditional standard deviation of the monthly returns given prior information.
Table 6: VAR Model of Stock-Price Volatilities
Dependent variable: Schwert share price volatility index
  Australia Germany Japan Singapore UK US
Australia 2.91 1.37 0.66 1.40 1.38 1.10
Germany 3.88 2.91 2.41 6.04 2.96 2.79
Japan 2.53 2.23 3.05 5.76 1.70 1.62
Singapore 3.37 1.31 4.22 3.56 2.82 1.54
UK 4.40 3.91 2.73 3.44 2.54 3.34
US 2.64 1.43 3.36 1.58 3.19 2.98

Notes: Reproduced from Panel B of Table 1 in McNelis (1993). Data is end month share price indexes over the period, January 1982 to March 1992. The VAR model regresses the volatility measure for each country on 6 lags of itself and 6 lags of the other countries' measure. If the joint significance of the 6 lags of a country are insignificant then the conclusion is that past values of that country do not contain significant information about predicting the dependent variable. The F-test statistic is reported in the table above: Critical Values on Zero Restrictions: F(6/56) = 2.27(5%); 3.08(1%).

If foreign share markets influence Australian share markets then they can also influence Australian activity directly and/or indirectly through their effect on investment in Australia.[22] The direct effect is through the investment by foreign-owned domestic companies or subsidiaries operating in Australia. If the share price of the parent company is high because of high profits then the subsidiary may have greater access to low cost retained earnings of the parent company.[23]

The indirect effect of the foreign share market is via its influence on the Australian share market. This effect contains at least three inter-related transmission mechanisms. The first mechanism follows from Tobin's investment theory or ‘q’ theory.[24] This predicts that firms will invest if the replacement cost of the capital stock is less than the value of the firm. The second is a cost of funds argument. Domestic firms find the cost of equity falls with higher general share prices leading to an expansion in investment and output. The third is an expectations argument. Expectations of greater world activity increase the valuation of domestic companies and share prices. Coincident with the expectation of greater activity is the need for greater investment to meet the expected higher demand.

Using a ‘benchmark’ model based on the Gruen and Shuetrim (1994) model of Australian GDP, we introduce domestic and foreign share market variables to investigate their impact on the model. The appropriate share market variable to include depends on the transmission mechanism which it represents. Share market variables reflecting the first two transmission mechanisms set out above are similar but not identical. Barro (1990) argues the ratio of the share price index to the private investment deflator is a good measure of Tobin's ‘q’ for an economy. By contrast, Fama (1990) uses share market returns deflated by the consumer price index to predict output growth. Similar to Fama's approach, we construct the accumulation share price index (which incorporates dividends), deflated by the GDP deflator. The GDP deflator was chosen because it allows the share price of the firm to be expressed in terms of the firm's output price. The real share market price variable was found to be a trend stationary process and was therefore detrended. The detrending is theoretically appealing as it is expected that the cycle in the real share price and not its trend level will affect the cycle in activity.

Figure 6 shows the detrended real share price for Australia, the US and a composite world index. We see that the three series move closely together. However, the world series deviates substantially from the Australian and US series following the 1987 sell-off in share prices due to the long lag before the Japanese share market was also sold-off in January 1990.

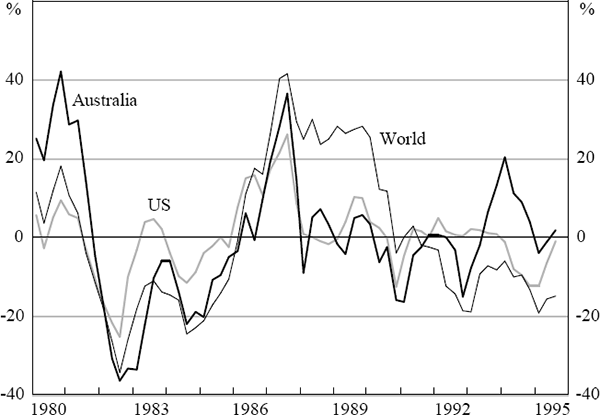
Figure 6: Detrended Real Share Prices
Figure 6: Detrended Real Share Prices

Note: The world real share price is the nominal world accumulation share price index deflated by the G7 deflator.

The benchmark model is reported in Table 7 using US GDP as the measure of foreign activity.[25] The US model was chosen over the OECD or export-market models as it performs substantially better. Models 1 to 3 add to the benchmark model the share market variables for Australia and the US. The models all perform better than the benchmark model in terms of increasing the explanatory power as measured by 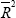Inline Equation. In model 1 we add the Australian real share price and find it has a significant and large, positive impact on Australian activity. A permanent 1 standard deviation increase in the real share price (around 17 per cent), increases GDP in the short run by around 0.32 of a percentage point and by around 1.26 percentage points in the long run. The addition to the benchmark model of the US real share price in model 2 provides similar results.[26] However, the long-run relationship between Australian and US GDP is less well defined.

Table 7: The Real Share Price and Australian Activity(a)
(1981:Q3–1995:Q3)
Dependent variable: Log change in Australian GDP
  Lag
 		 Benchmark model (1)
 		 (2)
 		 (3)
 
Constant   0.286*
(2.18)		 0.331**
(2.73)		 0.149
(1.11)		 0.303#
(1.90)
Australian GDP 1
 		 −0.287**
(−4.21)		 −0.257**
(−4.07)		 −0.205**
(−2.87)		 −0.246**
(−3.33)
US GDP 1
 		 0.345**
(4.44)		 0.300**
(4.13)		 0.254**
(3.12)		 0.289**
(3.52)
Real cash rate(b) 2 to 6
 		 −0.157**
{0.009}		 −0.149**
{0.006}		 −0.193**
{0.001}		 −0.156*
{0.034}
US GDP
(log change)		 0
 		 0.452**
(3.17)		 0.440**
(3.36)		 0.375**
(2.72)		 0.428**
(3.09)
Real share price
Australia 1
 		   0.019**
(3.17)		   0.017#
(1.74)
United States 1
 		     0.030*
(2.58)		 0.005
(0.283)
Long-run relationship
Foreign GDP   1.203 1.169 1.237 1.177
Joint significance of US and Australian share markets variables 4.97*
{0.011}
Diagnostics of residuals
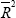Inline Equation   0.527 0.603 0.577 0.595
LM (1)(c)   2.61
{0.106}		 0.253
{0.615}		 0.101
{0.750}		 0.194
{0.659}
Standard error of equation   0.006 0.006 0.006 0.006
DW   1.59 1.85 1.90 1.87

Notes: (a) Each model was initially estimated with 4 lags of the short-run variables. Insignificant variables were then eliminated following individual exclusion tests. Finally, all the eliminated variables were tested for joint significance and rejected. Numbers in parentheses () are t-statistics and numbers in brackets {} are probability values for the joint test that all the lags can be excluded. The distribution of the t-statistics on the level variables in the models lies between a N(0, 1) and a Dickey Fuller distribution (see Kremers et al. 1992). **, *, and # denote significance at the 1%, 5%, and 10% levels respectively.
(b) Real cash rate reported as the sum of the coefficients multiplied by 100.
(c) LM (1) is a Lagrange multiplier test for first order autocorrelation.

Models 1 and 2 cannot separately identify the influence of the Australian and US share markets on output. In model 3 both share market variables are included. Evident from their joint significance, the US and Australian real share price variables contain information concerning Australian activity. Once the Australian share market variable is included, the US share market variable becomes insignificant. This suggests that the Australian variable contains all the relevant information present in the US variable plus some additional information. This result, along with the McNelis (1993) finding of ‘causality’ between the US and Australian share markets, is intuitively appealing. It is consistent with the idea that the US share market influences the Australian share market and, thereby, Australian activity, and that the Australian share market contains information not present in the US market which is uniquely relevant to Australian activity.

From models 2 and 3 we can determine the influence of the US share market on Australian activity. Model 3 could be used to identify the direct impact of the US share market on activity. However, the indirect effect via its influence on the Australian share market cannot be identified. Model 2 provides an estimate of the sum of the direct and indirect US influences.

The contributions to Australia's cycle in GDP from the US share market, the real cash rate and foreign activity using model 2 are shown in Figures 7a and 7b. We see that at times the foreign share market has a sizeable impact on GDP growth; up to 2 percentage points on a four-quarter-ended basis. In the bottom panel, a slightly larger impact stems from the real cash rate. The top panel of Figure 7b shows the contribution due to foreign growth. It is evident that while the contribution of the US share market to the cycle in Australian GDP has been smaller than that of the real cash rate or foreign activity, the contribution has been large at times and often leads the cycle.

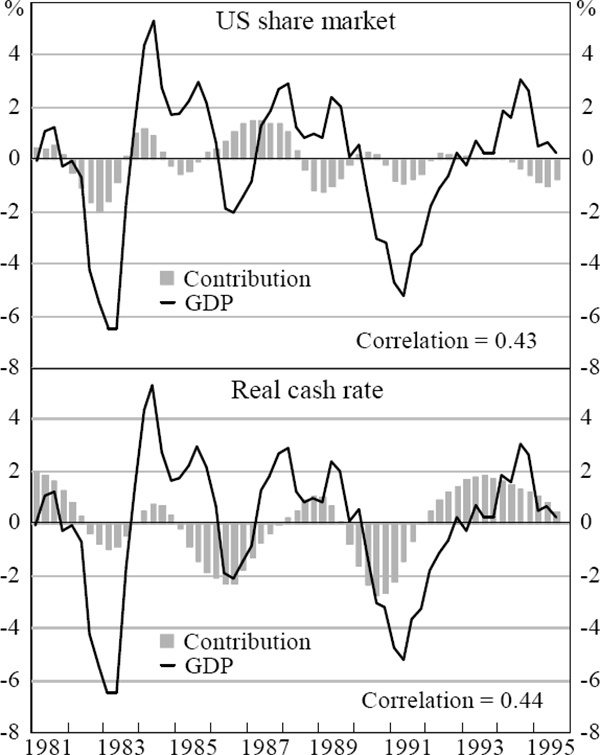
Figure 7a: Contributions to Australian Activity
Four-quarter-ended percentage change
Figure 7a: Contributions to Australian Activity

Notes: The GDP growth rate is the de-meaned four-quarter-ended growth in GDP. The contributions are calculated as follows. Predicted values for GDP growth are calculated using the actual values of the exogenous variables and the predicted level of GDP. Predicted values are also calculated holding a particular exogenous variable to its sample average growth rate or level over the entire sample. The contribution of that exogenous variable is then the difference between these predicted values.

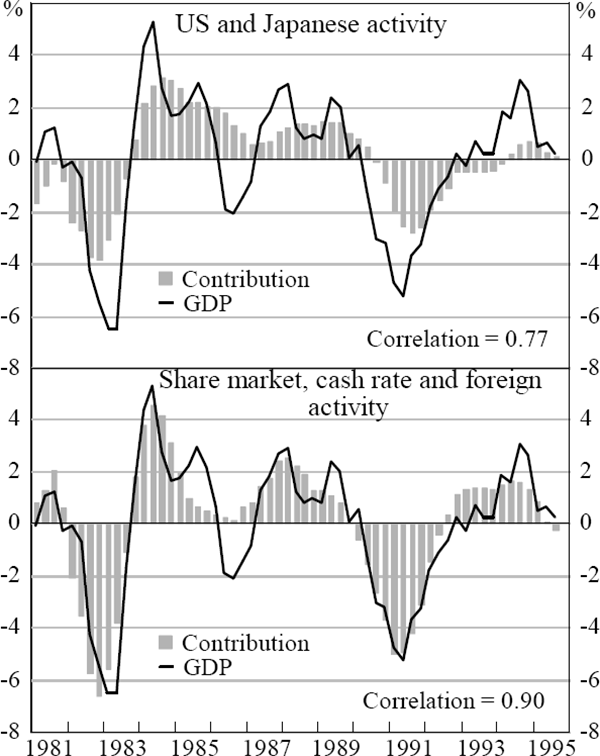
Figure 7b: Contributions to the Business Cycles
Four-quarter-ended percentage change
Figure 7b: Contributions to the Business Cycles

Notes: The GDP growth rate is the de-meaned four-quarter-ended growth in GDP. The contributions are calculated as follows. Predicted values for GDP growth are calculated using the actual values of the exogenous variables and the predicted level of GDP. Predicted values are also calculated holding a particular exogenous variable to its sample average growth rate or level over the entire sample. The contribution of that exogenous variable is then the difference between these predicted values.

Finally, we should notice that even though the inclusion of the share market variable increases the explanatory power of the benchmark model substantially, the short and long-run coefficients on foreign activity are not significantly reduced. It appears, therefore, that while the share market may help explain domestic activity, and may also help explain the correlation in business cycles, the foreign demand variable in the benchmark model is not simply a ‘proxy’ for a missing share market variable.

Footnotes

Under conditions of imperfect information, firms with higher net worth will, other things equal, have easier access to external funding. For example, see Gertler (1988) for a survey of the influences of financial factors on activity. See also Lowe and Rohling (1993) Mills, Morling and Tease (1994). [21]

While there may well be other avenues for share prices to effect domestic activity, such as a wealth effect on consumption, these other avenues appear minor and are not pursued. [22]

See Fazzari, Hubbard and Petersen (1988) and Froot and Stein (1991). [23]

See Tobin (1969). [24]

Over the longer sample, the ‘benchmark’ model differs slightly from that in Gruen and Shuetrim (1994). The Southern Oscillation Index is now insignificant. After testing for individual and joint significance, the terms of trade and real exchange rate were also eliminated from the benchmark model in a stepwise fashion. Consistent with the Gruen and Shuetrim model, a trend variable is also insignificant. [25]

The short and long-run impact on the level of Australian GDP of a permanent 1 standard deviation increase (around 9 per cent) in the US real share price are 0.27 and 1.31 percentage points. [26]