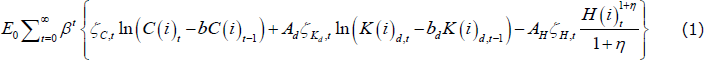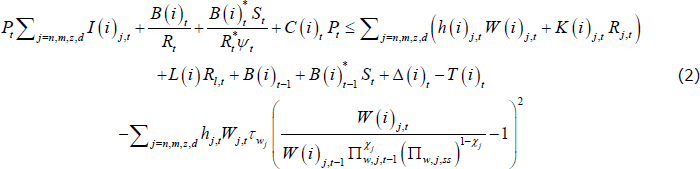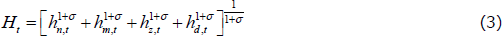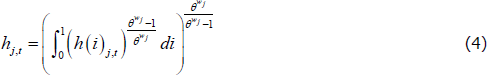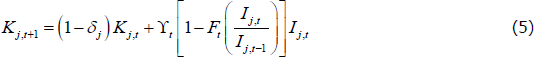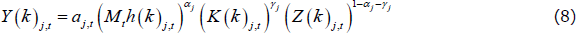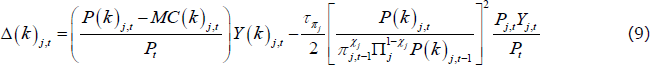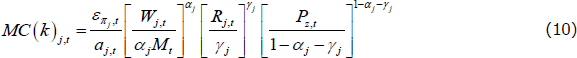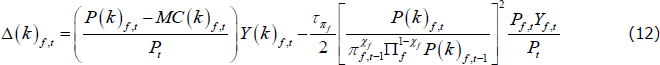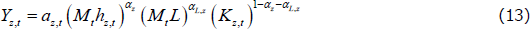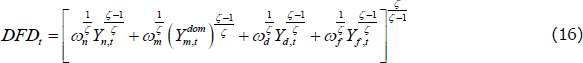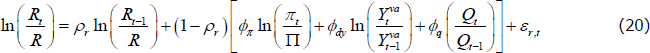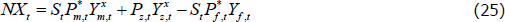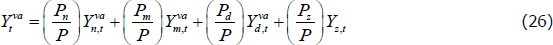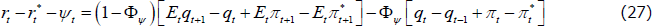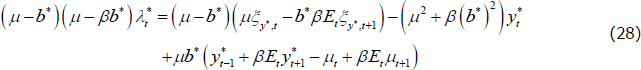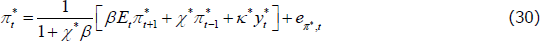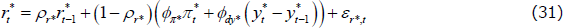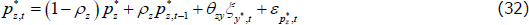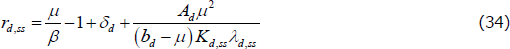RDP 2018-04: DSGE Reno: Adding a Housing Block to a Small Open Economy Model 2. The Model
April 2018
- Download the Paper 1,668KB
In this section, we provide a brief overview of the structure of the multi-sector model. We then present the household decision problem, an overview of the different sectors of the economy, and a brief discussion of the world economy model. We explain the accounting identities and the aggregation of the various sectors into the economy-wide aggregates. To build intuition for the economics behind the utility function specification, we also explore the steady-state properties of the housing sector using some partial-equilibrium comparative statics.
2.1 Overview
The multi-sector model we propose is a standard small open economy, sticky price, DSGE model. It represents an extension of the main DSGE model used by the Reserve Bank of Australia for policy analysis. The structure of the economy and the flows of real goods and services are shown in Figure 2.

The small open economy – Australia – consists of households and firms that produce and consume in six distinct sectors. There are five intermediate goods and services-producing sectors: resource (mining), non-resource tradeable (manufacturing and agriculture), non-tradeable excluding housing, housing, and imported goods and services. The resource sector is modelled as perfectly competitive and takes the world price of the resource good as given. The remaining sectors are monopolistically competitive and have some power to choose the prices of the goods they sell. Price changes for monopolistically competitive firms are subject to Rotemberg (1982) style price adjustment costs. Wages in all domestic production sectors face similar adjustment costs. These adjustment costs generate price and wage stickiness in the model. Intermediate goods and services, produced domestically and imported from abroad, are combined into final goods by the perfectly competitive final goods sector, which provides goods for household and government consumption as well as business investment.
Households derive utility from consumption of the composite final good and from the level of the housing stock in the economy, and derive disutility from labour supplied to firms in the four domestic intermediate production sectors. Households earn wages from labour supplied, rents from their ownership of capital, and dividends from ownership of firms. Households may also purchase domestic and foreign nominal bonds.
Monetary policy in the model follows a Taylor-type rule that responds more than one-for-one to changes in inflation and positively to changes in real GDP growth. Fiscal policy is assumed passive with lump-sum taxation.
The world economy is significantly less detailed. The world is modelled as a two sector closed economy version of the model just described, and also features price stickiness. It purchases resources and tradeable goods from Australia, and sells tradeable goods to Australia. Households in the world economy may invest in their own and Australian nominal bonds.
2.2 The Household's Decision
The household's decision problem is to choose consumption, hours worked, and the level of the housing stock to maximise expected utility subject to a budget constraint. In particular, the household consumes a composite consumption good that is a constant elasticity of substitution (CES) aggregate of all intermediate goods, including housing services. In addition, the households also gain utility directly from the level of the housing stock in the economy. Therefore, housing-related quantities enter the utility function in two different ways. This specification provides us with a demand for housing services and a separate supply of housing stock. One can think of this as separating the household's need to consume housing services from their preference for home ownership. These two quantities, in principle, can move somewhat independently of one another.
Formally, we assume that there is a continuum of identical households indexed by i and distributed uniformly on the unit interval. The households choose a sequence of consumption, C(i)t, hours worked, H(i)t, and housing capital stock, K(i)d,t, to maximise the following utility function:
subject to the following budget constraint:
Households' preferences are subject to three autoregressive preference shocks ζC,t, 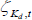 and ζH,t and exhibit habit persistence in both the level of consumption, b, and the level of the housing stock, bd. Ad and AH are normalising constants that pin down the steady state of the model so that it matches the data. Total hours worked Ht is an index composed of the hours allocated by households to each different production sector:
and ζH,t and exhibit habit persistence in both the level of consumption, b, and the level of the housing stock, bd. Ad and AH are normalising constants that pin down the steady state of the model so that it matches the data. Total hours worked Ht is an index composed of the hours allocated by households to each different production sector:
In the budget constraint, h(i)j,t and W(i)j,t denote hours worked and the nominal wage of household i in sector j; hj,t and Wj,t are average hours and wages in sector j, respectively; 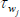 is the wage adjustment cost in sector j; I(i)j,t and K(i)j,t are investment and capital, respectively, by household i in sector j; L is land; Δ(i)t and T(i)t are dividends and lump-sum taxes, respectively; Pt is the price level; St is the nominal exchange rate; ψt is a risk premium shock; B(i)t is the amount household i has invested in one-period risk-free nominal bonds; Rt is the nominal interest rate factor, and starred variables denote foreign quantities. The four domestic production sectors of the economy are denoted j = {n, m, z, d}, where n is non-tradeable excluding housing, m is non-resource tradeable, z is resources, and d is the housing (dwelling) sector.[7]
is the wage adjustment cost in sector j; I(i)j,t and K(i)j,t are investment and capital, respectively, by household i in sector j; L is land; Δ(i)t and T(i)t are dividends and lump-sum taxes, respectively; Pt is the price level; St is the nominal exchange rate; ψt is a risk premium shock; B(i)t is the amount household i has invested in one-period risk-free nominal bonds; Rt is the nominal interest rate factor, and starred variables denote foreign quantities. The four domestic production sectors of the economy are denoted j = {n, m, z, d}, where n is non-tradeable excluding housing, m is non-resource tradeable, z is resources, and d is the housing (dwelling) sector.[7]
The final term of the budget constraint captures Rotemberg (1982) style wage adjustment costs with indexation, which we use to introduce wage stickiness into the model. The specification follows Burgess et al (2013). We assume that workers have heterogeneous skills. Firms purchase a bundle of labour from households that includes workers of all types:
Workers, therefore, have some bargaining power over their wages and take into consideration overall demand for labour and average wages in the market when negotiating. In addition, wage changes may be indexed to a combination of previous period's sectoral wage growth Πw,j,t − 1, and steady-state sectoral wage growth Πw,j,ss. The parameter  determines the degree of substitutability among differentiated labour inputs in sector j.
determines the degree of substitutability among differentiated labour inputs in sector j.
Households are homogenous, so individual consumption and saving decisions mirror the aggregated decisions. The aggregate capital stock of each sector in the economy evolves according to the following law of motion:
where δj is the depreciation rate of capital in sector j ∈ {n, m, z, d}, Ft (·) is the capital adjustment cost, and ϒt is an autoregressive investment adjustment cost shock.[8]
2.3 Sectoral Production
There are three monopolistically competitive intermediate goods-producing sectors in the model, which all share the same underlying structure: the non-tradeable excluding housing, the non-resource tradeable and the housing sectors. Additionally, there is a monopolistically competitive intermediate import sector and a perfectly competitive resource sector. The perfectly competitive final goods sector assembles the domestically sold output of these five sectors.
2.3.1 Intermediate monopolistically competitive goods and services producers
In the three monopolistically competitive producing sectors, we assume that there is a continuum of firms, indexed by k, that produce different intermediate goods using three factors: capital, labour, and the resource good. The firms sell their output to a wholesaler, who combines the differentiated intermediate goods into a homogenous good for sale in the final goods and services sector using a CES production technology:
where Y(k)j,t is output of firm k, and 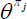 governs the degree of substitutability among the different intermediate goods in sector j. The demand for each firm's output is given by:
governs the degree of substitutability among the different intermediate goods in sector j. The demand for each firm's output is given by:
Given the demand for a firm's product, the firm must choose the amount of capital and labour to hire, the price of their product, and the amount of resources to use in production. These decisions are mostly the same across the three intermediate domestic goods-producing sectors except for the tradeable sector, where firms additionally must choose how much to export and at what price. The decision in this case, though, mirrors the decision for the domestic market. Therefore, in the interest of space, we present only the decision problem for firms selling in the domestic market.[9]
Each intermediate firm produces a unique variety of goods and services using the following production function:
where h(k)j,t, K(k)j,t, and Z(k)j,t are the quantities of each input used by the kth firm, aj,t is a sector-specific autoregressive technology shock in sector j, αj and γj are the labour and capital shares, respectively, in sector j, and Mt is an aggregate permanent labour-augmenting technology shock that follows a process made up of two components: a random walk with drift component and an autoregressive component.[10] A firm's objective is to choose a sequence of factor inputs and prices to maximise the expected discounted value of profits into the infinite future, subject to demand for Y(k)j,t, where a firm's real profit in period t is given by:
The firm's pricing decision is subject to a quadratic price adjustment cost, which is scaled by 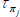 .
.
In addition, firms may index their price to a combination of πj,t − 1 and Πj, which are the previous period's sector-specific inflation rate and the steady-state sectoral inflation rate, respectively.
The MC(k)j,t are the marginal costs for firm k in sector j, which take the form:
where 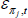 is a white noise mark-up shock that increases marginal costs in sector j, and Pz,t is the domestic currency price of commodities.
is a white noise mark-up shock that increases marginal costs in sector j, and Pz,t is the domestic currency price of commodities.
In the case of housing services production, the intermediate producer can be thought of as professional property developers and managers. These developer/managers rent housing stock from households and combine it with labour and resources inputs to produce housing services. The housing services are then sold to the final goods producers to be combined with all other intermediates, which in turn are sold on to households as part of the CES consumption bundle. The price for housing services, therefore, reflects a composite of rents, new dwelling prices and real estate services, which are the main housing components that are included in the CPI. As discussed in the introduction, this represents a fundamental difference compared to Iacoviello-type models, where the price of housing is a measure of the sale price of new and existing real estate.
2.3.2 Imports, resources and final goods sectors
2.3.2.1 Imports sector
Firms in the imports sector, f, import differentiated goods and services from overseas, which they sell to wholesalers as the other intermediate sectors do. The marginal cost of importing goods and services by firm k is given by:
where 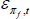 is an autoregressive mark-up shock. The firms seek to maximise expected profits by choosing a sequence of prices and the amount of goods and services to import, subject to demand for Y(k)f,t and quadratic price adjustment costs. Real profits in the sector are thus given by:
is an autoregressive mark-up shock. The firms seek to maximise expected profits by choosing a sequence of prices and the amount of goods and services to import, subject to demand for Y(k)f,t and quadratic price adjustment costs. Real profits in the sector are thus given by:
2.3.2.2 Resource sector
The resource sector is perfectly competitive and produces a homogeneous resource good. The resource good production function is given by:
where the stationary sector-specific technology shock takes on the same functional form as in the other sectors. The resource firms take the price as given and choose capital and labour to maximise profit, which is given by:
Following RSH, we assume that the law of one price holds for resources in the long run. However, we allow for a delay in the short-term pass-through of global resource price movements to the domestic price. This assumption captures the fact that a fraction of the resources exported from Australia are priced using contracts, which are only revised periodically, and that firms often hedge their foreign currency exposures. Therefore, the domestic price is assumed to follow:
where 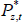 denotes the price in foreign currency terms, and St is the nominal exchange rate.
denotes the price in foreign currency terms, and St is the nominal exchange rate.
2.3.2.3 Finals goods sector
The final goods sector purchases the homogenous composite goods from the wholesalers and assembles them into final goods using the following production function:
where ωn + ωm + ωd + ωf = 1 and govern the shares of each sector's output in the final domestic good, 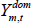 stands for tradeable goods sold domestically, and DFDt is domestic final demand. Profit maximisation by the final goods producers implies the following aggregate price index:
stands for tradeable goods sold domestically, and DFDt is domestic final demand. Profit maximisation by the final goods producers implies the following aggregate price index:
2.4 Fiscal and Monetary Policy
Following RSH, fiscal policy is assumed to be passive. The government issues bonds and raises lump-sum taxes for expenditures. The government's budget constraint is given by:
Public demand, Gt, is assumed exogenous and takes the following form:
where g determines public demand's steady-state share of GDP. The lump-sum taxes assumption implies that Ricardian equivalence holds in the model, meaning that the timing of taxation does not affect households' and firms' decisions. In addition, we assume that government debt is held in zero net supply.
Monetary policy is assumed to be active and follows a Taylor-type rule. Therefore, short-term nominal interest rates are set according to:
where πt is the CPI inflation rate, 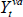 is real GDP (value added measure), Qt is the real exchange rate, ρr governs the degree of interest rate smoothing, and εr,t is a monetary policy shock. The parameters R and Π are steady-state interest rates and inflation, respectively.
is real GDP (value added measure), Qt is the real exchange rate, ρr governs the degree of interest rate smoothing, and εr,t is a monetary policy shock. The parameters R and Π are steady-state interest rates and inflation, respectively.
2.5 Market Clearing and the Current Account
The goods market clearing conditions are:
The first condition requires all tradeable goods and services produced to be sold domestically or abroad. The second condition requires all resource goods produced to be sold abroad or to the three intermediate production sectors. The third condition says that all final goods must be sold to consumers, the government or invested.
The net foreign asset position of the economy is given by the current account:
where NXt is nominal net exports. Net exports in this case are equal to the sum of the exported traded goods and the traded resources, less imports:
In addition, the non-tradeable, tradeable, and housing sectors all use resources as inputs. This introduces a wedge between the production and the value added in these sectors. Therefore, the relevant concept of GDP in this case requires a value-added computation. Following RSH, we do this calculation by subtracting out the value of the resource input in each intermediate sector using steady-state prices Pj. For example, 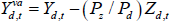 . Therefore, real GDP is defined as:
. Therefore, real GDP is defined as:
Finally, the following uncovered interest rate parity (UIP) condition holds in the log-linearised model:
2.6 The Foreign Economy
The foreign economy is modelled as a standard closed economy sticky-price model. The log-linearised equations of this model are given by:
where Equations (28) and (29) together form the IS relationship, Equation (30) is the Phillips curve, and Equation (31) is the monetary policy rule. The foreign demand shock, 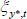 , and the foreign mark-up shock,
, and the foreign mark-up shock,  , are assumed to follow autoregressive processes, while the remaining shocks are assumed to be white noise.
, are assumed to follow autoregressive processes, while the remaining shocks are assumed to be white noise.
Commodity prices are assumed to follow an exogenous autoregressive process, which depends on its own shock and on the foreign demand shock:
where 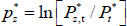 is the relative price of the resource good in terms of the foreign currency.
is the relative price of the resource good in terms of the foreign currency.
2.7 Understanding Housing Capital Stock in the Utility Function
Adding the housing stock to the utility function has implications for the steady-state and dynamic predictions of the model. To build intuition for these effects, we present some partial equilibrium analysis using the steady-state relationships. This analysis allows us to look at the supply and demand relationships for the housing stock implied by the model and to do some basic curve shifting exercises. The dynamic general equilibrium predictions of the model are discussed in Sections 4 and 5.
The addition of the housing stock to the utility function primarily affects the supply of housing in the housing capital factor market. The capital factor markets in the model are perfectly competitive and can be illustrated using supply and demand schedules. The suppliers of the housing stock in this economy are the households. Supplying housing is one way in which households can save, and the stock of housing generates utility for the households. The demand for the housing stock comes from the firms in the housing services sector. These firms combine the housing stock with labour and resource goods to produce housing services, which are sold to the final goods producers and eventually to households for consumption. Therefore, the households' supply of housing may be thought of as the proportion of their portfolios invested in residential real estate.
To understand the effect of our preference assumption, we first discuss the households' supply of capital to any sector j when capital is not included in the utility function. In this case, the steady-state supply of capital is a fixed share of household saving that is determined solely by its return.
The return is pinned down by aggregate productivity, the household's discount rate, and the depreciation rate:
where μ is the steady-state labour-augmenting productivity growth rate. This relationship implies that households are willing to supply whatever the amount of capital the market demands at the current returns, and therefore, it generates a perfectly elastic supply schedule (represented by the horizontal line in Figure 3). The demand for capital comes from the marginal productivity of capital implied by the production technology. The production technology is Cobb-Douglas in all sectors so there are diminishing returns to capital, which give rise to the standard downward sloping demand schedule (Figure 3).
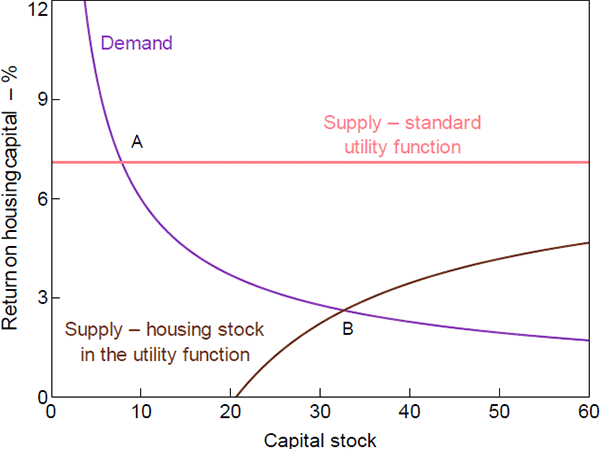
When we include the housing stock in the utility function, the household's supply schedule for housing capital is changed. Since households derive utility from holding the housing capital stock, they invest more in this sector. In doing so, they weigh the utility they get from the current and future level of the housing capital stock with the utility of consumption, which gives rise to the following supply schedule:
where λd,ss is the steady-state ‘shadow’ price of housing (i.e. the Lagrange multiplier associated with housing capital from the household's constrained optimisation problem), which is equivalent to the marginal utility of consumption.
Equation (34) implies an upward sloping relationship since gross steady-state productivity growth is assumed greater than one and habit persistence is less than one (Figure 3). Intuitively, the supply curve slopes upward because of the assumption of diminishing marginal utility of the capital stock in the preference specification. Households are no longer willing to supply whatever amount of capital is demanded because higher levels of capital stock mean lower marginal utility, and therefore a higher rate of return on the housing capital stock is now required to induce more capital supply.
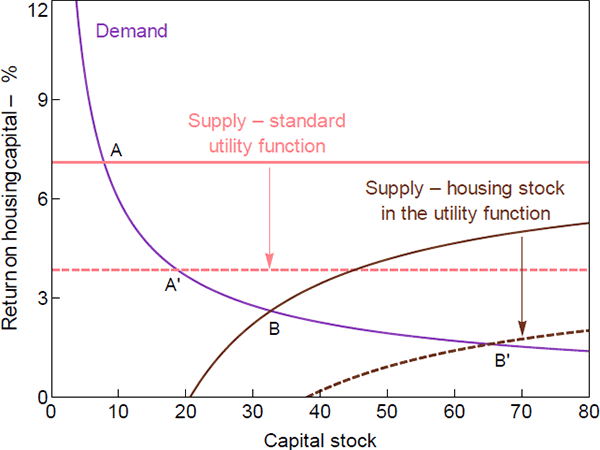
Moreover, when housing is included in the utility function, the supply curve for the housing capital stock is shifted to the right, relative to the case without housing in the utility function.[11] This reflects the fact that households gain utility from holding the capital and therefore require lower returns in steady state. Consequently, the steady-state rate of return is lower, while the steady-state level of housing is higher (point B in Figure 3 instead of point A). This higher steady-state level of housing turns out to be a key feature of the model, which helps it to match the data.
The differences in the supply curves, and their intersections with the demand curve, imply different responses of housing investment to changes in steady-state real interest rates. Figure 4 shows the predicted change in the capital stock for a decrease in the real interest rate due to an increase the household discount factor.[12] A fall in the steady-state real interest rate shifts the supply curve for the housing capital stock to the right. This shift results in a higher steady-state quantity of housing and pushes down the rate of return. Due to the different curvatures of the supply schedules, and the different initial conditions, the increase in housing is much larger in the case where the housing stock is included in the utility function, while the effect on the return is smaller (with the economy moving from point AꞋ to BꞋ in Figure 4 instead of from point A to B). Therefore, ceteris paribus, the same fall in interest rates generates a larger investment response when the housing stock is included in the household utility function, compared to the case when it is not included.
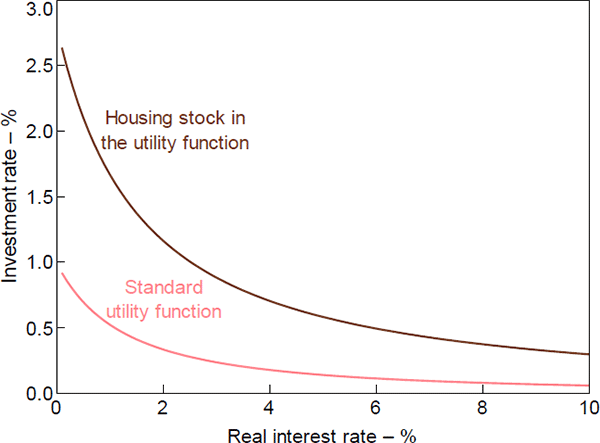
Figure 5 quantifies the difference in the investment response between the two cases for a range of steady-state values of the real interest rate. As the steady-state real interest rate falls, investment in the housing capital stock rises in both cases. However, the difference in the responses between the two cases increases as the interest rate approaches zero.
To summarise, in steady state, the assumption that the housing stock appears in the utility function implies that households hold more housing than would otherwise be the case, and that investment is more responsive to changes in the steady-state real interest rates. The increased sensitivity of housing investment to interest rates will carry over to the dynamic predictions of the model.
Footnotes
We follow RSH and assume that the risk premium shock, ψt, depends on the stock of foreign debt outstanding such that 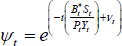 , where νt follows an autoregressive process.
[7]
, where νt follows an autoregressive process.
[7]
We assume that F is a function of the steady-state growth rate of labour-augmenting productivity, μ, such that 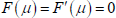 and
and 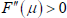 . In practice, we adopt the following function form:
. In practice, we adopt the following function form: 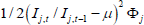 .
[8]
.
[8]
For a detailed description of each individual sector we direct the interested reader to RSH. [9]
Despite land being included in the model as part of the mining production function, we do not include land as a factor of production for the housing sector. Instead, to retain a close link to the original model and to the production of other non-tradeable goods, we choose the same functional form for the production function as that of the non-tradeable sector. Considering a fixed factor here is an interesting area to explore in future iterations of the model. [10]
The calibration parameters for this exercise are β = 0.95, μ = 1.008, bd = 0.15, Ad = 0.3, and λd = 1. [11]
In steady state, the real interest rate is pinned down by the household discount factor, β. That is, for a given value of the discount factor the steady-state real interest rate is such that households are indifferent between consumption today and consumption in the future. Increases in β correspond to decreases in the steady-state value of the real rate of interest since the value of future consumption rises relative to that of current consumption. [12]