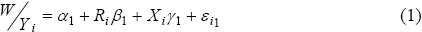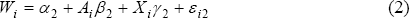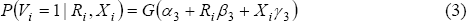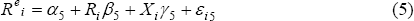RDP 2007-08: The Effect of the Australian Superannuation Guarantee on Household Saving Behaviour 2. Methodology

2.1 Identification Strategy

The aim is to estimate the effect of compulsory pension accounts on household wealth, retirement saving and the timing of retirement. Australia's system provides a natural experiment, as around 8 per cent of employees did not receive employer contributions to pension accounts in August 2002 (ABS 2003b). Therefore, households that received contributions can be compared with those that did not. The main exemptions from making compulsory contributions are for the employers of individuals earning less than $450 per month, those under 18 years of age who work less than 30 hours a week and certain jobs such as transport drivers, household employees and providers of child care in the home.^[6] Those who are not remunerated as employees, such as independent contractors and the self-employed, are also exempt. Finally, there may be a small percentage of employers who are avoiding making contributions despite being legally required to do so. Such employers are likely to be part of the underground economy, which the ABS (2003a) has estimated to be up to 2 per cent of GDP.

The key assumption underlying the identification strategy is that the decision of the Government and employers as to whether employees receive compulsory pension contributions is exogenous with respect to the employees' unobserved taste for saving, after controlling for covariates. This assumption is analogous to the 401(k) eligibility experiment of Poterba, Venti and Wise (1996) for the US, where it was assumed that employers choose whether their employees are eligible to contribute to a 401(k) plan. It is then possible to measure the effect of eligibility by comparing eligible employees to ineligible employees.

One way to test the validity of this identification strategy would be to compare the two groups before the introduction of compulsory pension accounts. Unfortunately, the data required to conduct such an analysis are not available for Australia. Instead, the subjective saving behaviour of both groups can be examined subsequent to the introduction of compulsory pension accounts. I find that the two groups have similar reasons for saving, horizons for saving and appetites for risk (see Appendix A, Table A1).^[7] However, if compulsory pension accounts were to fundamentally alter household saving behaviour, then comparing the two groups after the introduction of the accounts may not reflect their behaviour in the absence of such accounts.

The obvious violation of this identification assumption is the self-employment exemption. The self-employed may choose not to remunerate their household as ‘employees’ so as to avoid having to pay (and receive) compulsory pension contributions. This decision could be correlated with their unobserved taste for saving. I control for this in several ways: employers and own-account workers are excluded from the sample; and for persons who are employees of their own business, self-employment is included as a covariate and also interacted with the variable measuring whether or not households receive compulsory pension contributions.

2.2 Household Wealth

To measure the effect of compulsory pension accounts on wealth, I estimate the following regression:

where: 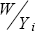is a measure of the wealth-to-income ratio; R_i is a dummy variable that takes the value 1 if household i receives compulsory pension contributions and 0 otherwise; X_i is a matrix of covariates; α₁,β₁ and γ₁ are parameters; and ε_i1 is an independent and identically distributed error term. I estimate the effect on the wealth-to-income ratio since compulsory contributions to pension accounts should be proportional to labour income. If compulsory contributions have a positive effect on the wealth-to-income ratio, then β₁>0. To reduce the influence of outliers in the wealth distribution on the estimates, median regression is used with bootstrapped standard errors.

To obtain an estimate of the effect of a marginal increase in compulsory pension account balances on household wealth, I estimate the following regression specification:

where: A_i is an estimate of the value of the compulsory pension account of household i; and β₂ is a parameter that measures the marginal effect of a dollar of saving in compulsory pension accounts on net wealth.

2.3 Voluntary Saving for Retirement

The decision of households to make voluntary contributions to their pension accounts can be modelled as a function of whether they receive compulsory pension account contributions:

where V_i takes the value 1 if household i makes voluntary contributions and 0 otherwise. Since V_i is a dummy variable, this regression is estimated as a probit model, where G is the standard normal cumulative distribution function. If β₃>0, there is a positive relationship between employers making compulsory contributions and employees making voluntary contributions (and vice versa if β₃<0). Since the magnitudes of the coefficients in a probit model have no straightforward economic interpretation, marginal effects (the estimated changes in the probability in response to changes in regressors) are reported.

The magnitude of the effect on voluntary saving for retirement can also be modelled using data on the share of income that households voluntarily contribute to their pension accounts as the regressand. In this case, V_i is continuous, but has a lower threshold of 0 per cent, since households cannot make withdrawals from their retirement accounts prior to retirement. Hence a tobit model is estimated and marginal effects are reported. If the marginal effect is greater than zero, then compulsory accounts result in employees making larger voluntary contributions.

2.4 Retirement Intentions

Since Australia's compulsory pension accounts policy has only been operating fully since 1992, it is too early to estimate the effect of compulsory accounts on retirement outcomes. Instead, to obtain some preliminary evidence, I estimate the effect on the retirement intentions of working household members aged over 45. There are two variables that can be analysed. First, it is possible to examine whether household members actually have an intended age of retirement:

where 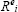takes the value 1 if at least one household member aged over 45 has an intended retirement age and 0 otherwise. Whether household members have an intended retirement age could be considered a partial indicator of the extent to which the household has engaged in retirement planning. If β₄>0, then compulsory pension accounts increase the likelihood that households have an intended age of retirement.

Second, the effect on the average intended age of retirement can be examined (for those households where at least one person specified an intended retirement age):

where 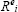is the average intended age of retirement of working household members over the age of 45. If β₅<0, then compulsory accounts encourage early retirement.

2.5 Controlling for Other Factors Affecting Saving

The aim is to develop a model of saving behaviour where, after controlling for covariates, the decision of the government and employers as to whether households receive compulsory pension contributions is exogenous with respect to households' unobserved taste for saving.^[8] Therefore, in the matrix of covariates, X_i, I include: household income, age, gender and health condition of the household head, which affect saving through the permanent-income and life-cycle hypotheses; whether someone in the household is self-employed; the industry in which the household head works, since institutional arrangements for pensions differ by industry; and the subjective job insecurity of the household head, to control for the fact that households not receiving pension contributions are more likely to be casually employed or on contract, and may have a greater precautionary motive for saving.

Other covariates typically used in wealth regressions are also included: the education level of the household head, as an indicator of human capital and possibly of financial sophistication; the marital status of the household head and family size, which can affect households' saving; location, which is an important explanator of property value; and the number of years the household head has spent in the workforce, which controls for households' exposure to pension account accumulation and income flows.^[9] Where a covariate is a continuous variable (such as age and income), it is specified using a quadratic form (with a linear and a squared term) to allow for potential non-linearities.

Footnotes

Some persons in the following occupations may not receive pension contributions: artists, painters, photographers, journalists, transport drivers, army reservists and child care and personal services workers in the home (who work for not more than 30 hours per week). [6]

Pence (2002) conducted a very similar exercise for 401(k) participants, but with the added advantage of access to subjective saving behaviour data that pre-dated the widespread adoption of 401(k)s. She concluded that participation in 401(k)s did not appear to fundamentally alter saving behaviour. [7]

Ideally, I would also model intra-household decisions, since the employment experiences and characteristics of different household members could affect household saving. However, many components of wealth have only been surveyed on a household basis, so the modelling of intra-household decisions is beyond the scope of this paper. [8]

See, for example, Poterba et al (1996) and Pence (2001). [9]