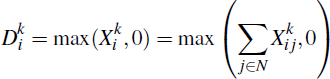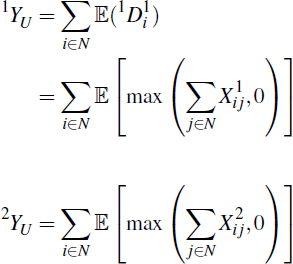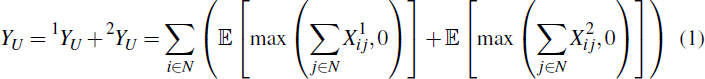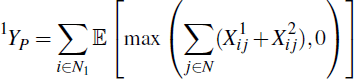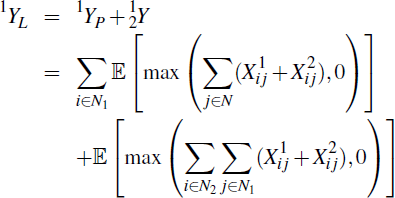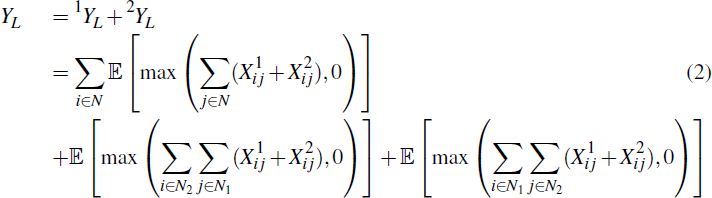RDP 2013-12: Central Counterparty Links and Clearing System Exposures 3. Model Framework

We extend the Duffie and Zhu (2011) framework to compare two scenarios: CCPs operating separately and CCPs operating under a link arrangement. The variable of interest is the expected value of aggregate CCP exposures in the system. Since CCPs must meet the obligations of defaulting participants and linked CCPs, the exposures of CCPs to their participants are determined by the net variation-margin obligations that participants have to each other.^[4] To the extent that collateral requirements accurately reflect underlying exposures, changes in exposures would flow through to a corresponding change in collateral requirements.

To isolate the effects of a link arrangement, the number of participants and the number and types of their positions remain constant across both scenarios. The simplest way to achieve this is to assume that every participant has positions with every other participant in each product, and that all bilateral positions are cleared centrally. This section starts by describing the two scenarios, before introducing the formal model, defining its key variables and outlining the main assumptions used to derive the analytical results presented in Section 4.

3.1 Participation and Positions

In the unlinked scenario, participants have full market access and join all CCPs. This could reflect participants' desire to be able to trade all products, access whichever market is offering better terms, or offer their clients the full range of clearing venues. The CCPs each clear one unique ‘product’.^[5] The aggregate CCP exposure is calculated by adding all of the expected obligations that the CCPs have to their participants.

In the linked scenario, each CCP can offer all products. Since it would be costly to maintain membership of all CCPs, each participant uses only one CCP for its full portfolio. Participant numbers in each CCP are treated as variables that always sum to the total number of participants in the unlinked case. All positions between participants using different CCPs go through the link, creating inter-CCP exposures. The aggregate CCP exposure in this case is the sum of these expected inter-CCP obligations and the obligations of the CCPs to their own participants.

In reality, not all participants are likely to be members of all CCPs in the unlinked case. However, modifying the model to allow for this would mean that the link gave participants more access to other participants than they had without the link. This would result in more positions being held, and therefore would not isolate the netting effects of a link arrangement.

In both cases, our approach to measuring aggregate exposure differs from that used by Anderson et al (2013), where participant exposures to CCPs are included. Our approach only accounts for CCPs' exposures, both to their participants and to each other when linked. Therefore, our conclusions about aggregate exposure will be indicative of required aggregate collateral, although we do not explicitly quantify collateral impacts. CCPs are regarded as prudent, regulated counterparties designed to withstand any individual participant default, even in extreme but plausible circumstances. Accordingly, it is uncommon for participants to receive collateral from CCPs. For this reason, participant exposure to CCPs is not included in our measure of aggregate exposure. However, collateral is required to manage inter-CCP exposures, owing to the large disruptions to the financial system that could be caused from a CCP defaulting on its obligations to a linked CCP. Hence these obligations count towards our measure of aggregate exposure.

3.2 Notation

3.2.1 Participant obligations

Consider N market participants trading K products through a CCP. For participants i and j, let 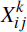represent the net obligation of i to j in product k that could accrue in the future over the close-out period. From the CCP's perspective, 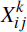is the obligation the CCP has to participant j in product k if participant i defaulted. For a close-out period similar in length to the frequency over which variation margining is calculated, 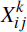can be thought of as a variation-margin obligation to the CCP. Ex ante, 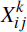is a random variable that depends on bilateral positions in product k and product k's price movements.

If 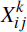> 0, participant i has a positive obligation to the CCP arising from its open interest with j in product k; accordingly, it would pay variation margin to the CCP for this position. A negative value of 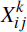implies that i should receive payment from the CCP on its positions with j in product k. The net obligation of participant i to a CCP clearing K products, however, is the sum across all j in N and k in K. Letting:

• X_ij be the net obligation of participant i to participant j in all products k
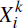• be the net obligation of participant i to all other participants j in product k
• X_i be the net obligation of participant i to all participants in all products.

We have:

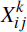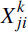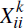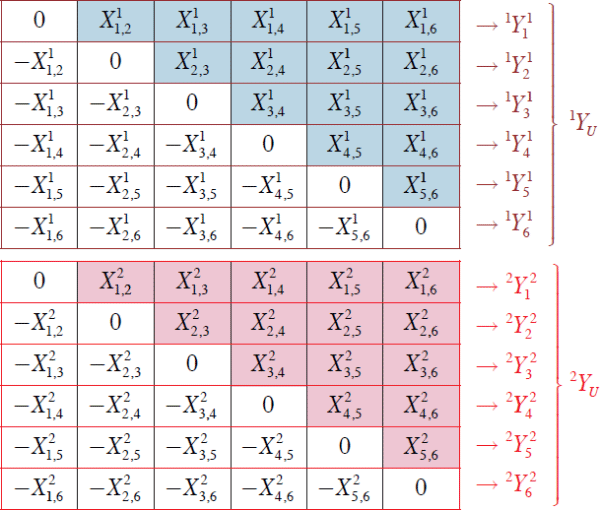
By definition, = − , and = 0. This means that the obligations of N participants in K products is characterised by KN(N −1)/2 random variables 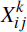, as shown in Figure 2 for the case of product k for N = 6.

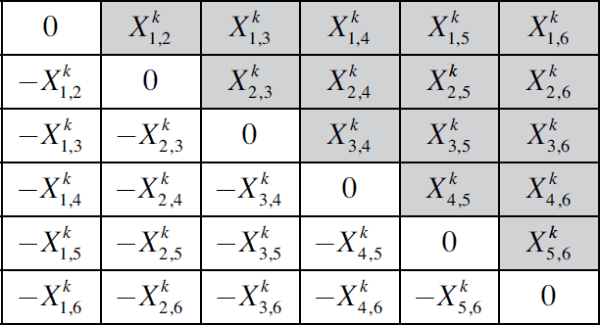
3.2.2 Default loss and exposure

When trades in a product are novated to a CCP, the CCP is responsible for meeting the obligations of any defaulting participants. If participant i defaults, the CCP's default loss in product k, , would be:

• the net of all participant i's obligations if > 0
• zero if 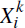≤ 0.

That is,

We consider an exposure to be the expected loss given default of a participant.^[6] This ignores the probability of the default occurring, which is consistent with common practice for setting collateral requirements (and which simplifies the analysis considerably). The CCP's exposure to participant i in product k is therefore:

Typically, the participant would be required to post collateral (initial margin) when entering the trade; this collateral is then drawn on by the CCP to cover the loss in the event of a default. The level of collateral required depends on the size of the default losses the CCP expects to have to cover.^[7] Accordingly, there is a clear relationship between expected losses given default and collateral requirements.

In the case of C CCPs, we denote as 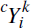the exposure of CCP c to participant i in product k (based on the corresponding loss given default 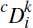). We denote as ^cY_P the exposure of CCP c to all participants in all of the products that it clears.

In the case of linked CCPs, we denote as 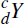the exposure of CCP c to CCP d in all products cleared across the link.

We denote as ^cY_U and ^cY_L the aggregate exposure of CCP c (in all products), both to participants and to other CCPs, in the unlinked and linked cases respectively. Y_U and Y_L denote the corresponding total exposures for all CCPs.

The following scenarios consider the case of two CCPs and two products (C = K = 2).

3.3 Aggregate CCP Exposure: Two Unlinked CCPs

Consider N participants, all members of two unlinked CCPs, CCP1 and CCP2. These CCPs each clear only one product – products 1 and 2, respectively. CCP1 is responsible for meeting the obligations of any defaulting participant in product 1, and CCP2 is responsible for meeting the obligations of any defaulting participant in product 2.

The expected exposure for CCP1,¹Y_U, is the sum of the expected losses given default in product 1 over all N participants, and similarly for CCP2:

The relationship of the CCP exposures to the participant obligations is illustrated in Figure 3. Because the CCPs are not linked, there is no inter-CCP exposure. Therefore, the total exposure across all CCPs in the unlinked case is:

3.4 Aggregate CCP Exposure: Two Linked CCPs

When CCP1 and CCP2 are linked, we assume participants consolidate their full portfolio into one CCP. Let there be N₁ and N₂ participants that consolidate in CCP1 and CCP2 respectively, where N₁ + N₂ = N. Since each participant's full portfolio (consisting of positions in products 1 and 2) is in a single CCP, participant obligations can be netted across products 1 and 2.

Under the link, CCP1 now faces two sources of exposure. The first arises from its N₁ participants' positions in products 1 and 2. CCP1 is responsible for meeting the obligations that any of its defaulting participants have, whether these be to other members of CCP1 or to members of CCP2. That is, where participant i is a member of CCP1, CCP1 is exposed to the net (if positive) of i's obligations across all j in N and all k in K (this is represented by the top three rows in Figure 4).

CCP1's exposure to its N₁ participants is therefore:

The second source of exposure faced by CCP1 in the linked case comes from CCP2. This is the expected loss that CCP1 would experience if CCP2 defaulted. In such an event, CCP1 would be responsible for meeting all obligations passing through the link, that is, all obligations of CCP2's members to CCP1's members. The default loss experienced by CCP1 would then be the net (if positive) of the obligations 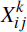across all i in N₂, j in N₁ and k in K (this is represented by the bottom left quadrant in Figure 4).

CCP1's exposure to CCP2 is therefore:

CCP1's overall exposure in the linked case is the sum of the exposure it has to its own participants and the exposure that it has to CCP2:

Similarly, CCP2 has two sources of exposure; that arising from its N₂ participants' obligations to all N participants (²Y_P, represented by the bottom three rows in Figure 4), and the inter-CCP exposure arising from CCP1's N₁ participants to its N₂ participants (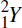, represented by the top right quadrant in Figure 4). CCP2's overall exposure in the linked case is:

The total exposure across all CCPs in the linked case is therefore:

The first term of Equation (2) reveals the additional intra-CCP netting that a link allows. Positive and negative obligations in products 1 and 2 can now offset each other because, for a given participant, they are concentrated in the one CCP. This reduces the CCPs' exposures to their own participants relative to the unlinked case. The second two terms, however, show the additional inter-CCP exposures introduced by the link. The key question posed by this paper is which of these two effects is larger. This determines whether CCP link arrangements introduce additional exposure, which will in turn need to be covered by more collateral. To answer this question, we now impose a distribution on participant-level obligations, .

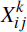3.5 Distributional Assumptions

Assuming a distribution for 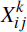with certain properties allows us to calculate aggregate exposures in the linked and unlinked cases. We will initially assume, for all i ≠ j, that the 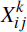are identically and normally distributed 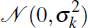, and are independent across i and j, but possibly correlated across k (with a constant correlation for all i and j).

The assumption that the 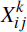are identically distributed imposes homogeneity on the participants. For each asset class, obligations between pairs of participants are assigned randomly from the same distribution, implying that any pair of participants is as likely as any other pair to have a bilateral obligation of a given size or sign, with no pair of participants more likely than any other pair to have a large (or small) bilateral obligation.^[8]

Although financial time series often display excess kurtosis, a normally distributed 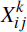can be taken as an acceptable first approximation. This is because the random variable of interest is ultimately the net obligations of participants to their CCP (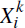), which are summations of the net obligations between participants (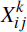). Under the central limit theorem, these summations (for a sufficiently large number of participants) lead to a normal distribution for 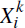regardless of the distribution of 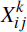, as long as they are identically distributed with finite variance and independent across i and j. Further, the 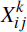are themselves net obligations between participants, and may represent the sums of various long and short positions in different products within contract class k. For these reasons, Section 4 will assume the normality of 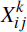in order to derive simple analytical solutions for Equations (1) and (2), while alternative distributions (as well as non-independence) will be considered using simulation methods in Section 5.

The distribution's zero mean relates to market efficiency, implying that participants' expected profits on their obligations to individual counterparties are each zero. While participants may have an expectation that their positions will be profitable, intermediate gains and losses are, in expectation, likely to be close to zero during a short close-out period. The variance for each product may reflect the volatility of price movements in the underlying products, but may also reflect the conscious decisions of participants to target desired levels of volatility, holding the number and combination of positions that will best achieve these levels.

The independence assumption implies that one participant's exposure is not related to another participant's exposure, and that a participant's exposure to one counterparty is not related to its exposure to another counterparty. This is particularly reasonable for centrally cleared markets, as the end-counterparty to each trade has virtually no effect on the value of the trade.

Participants' obligations in different products may be correlated. This may reflect covariance in the price movements of the products, or it may reflect the decisions of participants to hedge across products, with lower correlations reflecting greater hedging.

Footnotes

Variation margin is paid from a participant to a CCP (or from a CCP to a participant) to cover obligations arising from marking to market open positions with other participants. Typically, variation margin is payable daily. [4]

These may be the same product traded in different venues. This is the case for the current interoperability arrangements in place in European cash equity markets. For this reason, Section 4 assumes that CCPs clear the same products as a baseline case. [5]

This paper focuses on loss given default, in line with CCP margining practice. Modelling the actual probability of participant or CCP default is outside the scope of this paper. [6]

In accordance with relevant international standards (CPSS-IOSCO 2012), a CCP aims to be able to cover obligations arising in the event of the default of its largest one or two participants (by exposure) in ‘extreme but plausible’ market conditions, which are determined with reference to stress-test scenarios. For particularly extreme circumstances where initial margin does not cover the losses from a participant default, a CCP will generally hold additional resources. This typically takes the form of a default fund, which pools the CCP's own resources and participant contributions to cover these more extreme circumstances. [7]

Section 5 extends the model to allow for heterogeneity. [8]